Risk of a statistical procedure

A characteristic giving the mean loss of an experimenter in a problem of statistical decision making and thus defining the quality of the statistical procedure under consideration.

Suppose that one has to make a decision in a measurable decision space with respect to a parameter on the basis of a realization of a random variable with values in a sampling space , . Further, let the loss of a statistician caused by making the decision when the random variable follows the law be 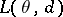, where is some loss function given on 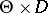. In this case, if the statistician uses a non-randomized decision function 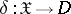in the problem of decision making, then as a characteristic of this function the function

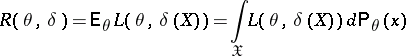is used. It is called the risk function or, simply, the risk, of the statistical procedure based on the decision function with respect to the loss .

The concept of risk allows one to introduce a partial order on the set of all non-randomized decision functions, since it is assumed that between two different decision functions 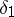and one should prefer if uniformly over all .

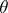If the decision function 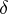is randomized, the risk of the statistical procedure is defined by the formula

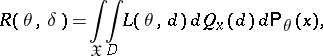where is the family of Markov transition probability distributions determining the randomization procedure.

References

[1]	E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1986)
[2]	N.N. Chentsov, "Statistical decision rules and optimal inference" , Amer. Math. Soc. (1982) (Translated from Russian)
[3]	A. Wald, "Statistical decision functions" , Wiley (1950)