Riemannian space, homogeneous
A Riemannian space 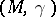 together with a transitive effective group
together with a transitive effective group  of motions (cf. Motion) on it. Let
of motions (cf. Motion) on it. Let 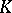 be the isotropy subgroup of a fixed point
be the isotropy subgroup of a fixed point 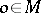 . Then the manifold
. Then the manifold  is identified with the quotient space
is identified with the quotient space 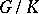 by the bijection
by the bijection 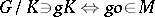 , and the Riemannian metric
, and the Riemannian metric 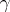 is considered as a
is considered as a 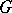 -invariant metric on
-invariant metric on  . Usually one assumes in addition that the group
. Usually one assumes in addition that the group 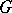 is closed in the complete group of motions. In this case the isotropy group
is closed in the complete group of motions. In this case the isotropy group  is compact.
is compact.
Let  be a compact subgroup of a Lie group
be a compact subgroup of a Lie group  that does not contain normal subgroups of
that does not contain normal subgroups of 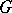 . Then the homogeneous space
. Then the homogeneous space 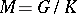 admits an invariant Riemannian metric
admits an invariant Riemannian metric 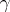 defined as follows. Let
defined as follows. Let 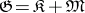 be a reductive structure in
be a reductive structure in 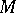 , i.e. a decomposition of the Lie algebra
, i.e. a decomposition of the Lie algebra  of
of  into the direct sum of the Lie algebra
into the direct sum of the Lie algebra 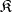 of
of 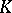 and a subspace
and a subspace  that is invariant under the adjoint representation
that is invariant under the adjoint representation 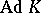 of
of 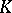 in
in 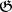 . The space
. The space  is naturally identified with the tangent space
is naturally identified with the tangent space  at the point
at the point 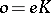 , and the isotropy representation of the group
, and the isotropy representation of the group 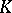 in
in 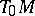 with the representation
with the representation  . Any
. Any 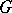 -invariant Riemannian metric
-invariant Riemannian metric 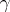 on
on 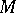 is obtained from some
is obtained from some  -invariant scalar product
-invariant scalar product  in
in 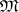 by translations from
by translations from  :
:
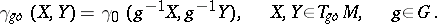 |
The existence of such a scalar product follows from the fact that the isotropy group 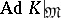 is compact.
is compact.
Any homogeneous Riemannian space locally isometric to a simply-connected homogeneous Riemannian space  is obtained from
is obtained from 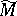 by factorization with respect to an arbitrary Clifford–Wolf discrete group of isometries (i.e. motions of the manifold
by factorization with respect to an arbitrary Clifford–Wolf discrete group of isometries (i.e. motions of the manifold 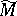 that displace all points by equal distances [2]).
that displace all points by equal distances [2]).
The best studied classes of homogeneous Riemannian spaces are the Riemannian symmetric spaces (cf. also Symmetric space); homogeneous Kähler spaces (cf. Kähler manifold) and homogeneous quaternionic spaces; isotropically-irreducible homogeneous Riemannian spaces (classified in [9], [10]); normal homogeneous Riemannian spaces, in which the scalar product 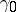 in
in 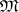 is defined by a non-degenerate symmetric
is defined by a non-degenerate symmetric  -invariant bilinear form on
-invariant bilinear form on  ; and naturally-reductive homogeneous Riemannian spaces, characterized by the fact that any geodesic in them is the trajectory of a one-parameter group of motions.
; and naturally-reductive homogeneous Riemannian spaces, characterized by the fact that any geodesic in them is the trajectory of a one-parameter group of motions.
The structure of homogeneous Riemannian spaces with different conditions on the curvature tensor is well studied. For instance, the classification of homogeneous Riemannian spaces of positive sectional curvature is known [5]. The structure of simply-transitive groups of motions of a homogeneous Riemannian space of non-positive curvature [8], of non-negative curvature and of non-negative Ricci curvature [4] has been described. A homogeneous Riemannian space with a solvable group of motions 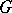 always has a non-positive scalar curvature
always has a non-positive scalar curvature 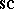 , and the case
, and the case 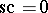 is possible only for locally Euclidean spaces. Any invariant Riemannian metric on a simply-connected homogeneous Riemannian space
is possible only for locally Euclidean spaces. Any invariant Riemannian metric on a simply-connected homogeneous Riemannian space 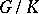 has non-positive scalar curvature if and only if
has non-positive scalar curvature if and only if 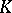 is a maximal compact subgroup of
is a maximal compact subgroup of 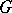 (see [4]).
(see [4]).
A homogeneous Riemannian space 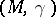 is called Einstein if its Ricci tensor
is called Einstein if its Ricci tensor 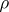 is proportional to the metric:
is proportional to the metric:  ,
, 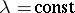 . The problem of the description of Einstein homogeneous Riemannian spaces has not yet been solved (1991). One knows a number of particular results. Let
. The problem of the description of Einstein homogeneous Riemannian spaces has not yet been solved (1991). One knows a number of particular results. Let 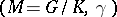 be an Einstein homogeneous Riemannian space of scalar curvature
be an Einstein homogeneous Riemannian space of scalar curvature 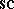 . 1) If
. 1) If  , then
, then 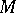 is a compact manifold. All such spaces have been described: a) if
is a compact manifold. All such spaces have been described: a) if 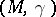 is a quaternionic space; b) if
is a quaternionic space; b) if  is diffeomorphic to a symmetric space of rank one; and c) for a certain class of naturally-reductive homogeneous Riemannian spaces (see [7]) and for isotropically-irreducible homogeneous Riemannian spaces (see [10]). 2) If
is diffeomorphic to a symmetric space of rank one; and c) for a certain class of naturally-reductive homogeneous Riemannian spaces (see [7]) and for isotropically-irreducible homogeneous Riemannian spaces (see [10]). 2) If 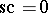 , then
, then 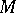 is a locally Euclidean space. 3) If
is a locally Euclidean space. 3) If 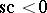 and
and 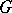 is unimodular (i.e. the determinant of its adjoint representation operator is equal to 1), then the group
is unimodular (i.e. the determinant of its adjoint representation operator is equal to 1), then the group 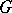 is semi-simple.
is semi-simple.
References
| [1] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1–2 , Interscience (1963–1968) |
| [2] | J.A. Wolf, "Spaces of constant curvature" , Publish or Perish (1977) |
| [3] | S. Helgason, "Differential geometry, Lie groups, and symmetric spaces" , Acad. Press (1978) |
| [4] | L. Berard Bergery, "Sur le courbure des métriques riemanniennes invariantes des groupes de Lie et des espaces homogènes" Ann. Sci. Ecole Norm. Sup. , 11 : 4 (1978) pp. 545–576 |
| [5] | L. Berard Bergery, "Les variétés riemanniennes simplement connexes de dimension impairé à courbure strictement positive" J. Math. Pures Appl. , 55 (1976) pp. 47–67 |
| [6] | G.R. Jensen, "Einstein metrics on principle fiber bundles" J. Dif. Geom. , 8 (1973) pp. 599–614 |
| [7] | J.E. d'Atri, W. Ziller, "Naturally reductive metrics and Einstein metrics on compact Lie groups" Mem. Amer. Math. Soc. , 18 (1979) pp. 1–72 |
| [8] | R. Azencott, E.N. Wilson, "Homogeneous manifolds with negative curvature II" Mem. Amer. Math. Soc. , 8 (1976) pp. 1–102 |
| [9] | O.V. Manturov, "Homogeneous Riemannian spaces with an irreducible rotation group" Trudy Sem. Vektor. i Tenzor. Anal. , 13 (1966) pp. 68–145 (In Russian) |
| [10] | J. Wolf, "The geometry and structure of isotropy irreducible homogeneous spaces" Acta Math. , 120 (1968) pp. 59–148 |
Comments
For a quite exhaustive treatment of Einstein manifolds see [a1], esp. Chapts. 7, 8.
Usually, an isometry of a Riemannian space is used as a synonym for motion, while the isometries in a Clifford–Wolf discrete group are known as Clifford translations, [2].
References
| [a1] | A.L. Besse, "Einstein manifolds" , Springer (1987) |
Riemannian space, homogeneous. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riemannian_space,_homogeneous&oldid=48562