Ricci identity

An identity expressing one of the properties of the Riemann tensor 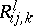(or ):

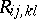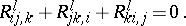For a covariant tensor 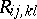the identity is of the form

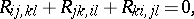i.e. cycling over the three first indices yields zero.

An identity which should be satisfied by the covariant derivatives of second order with respect to the metric tensor 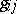of a Riemannian space , which differ only by the order of differentiation. If 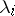is a tensor of valency 1 and 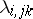is the covariant derivative of second order with respect to 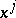and relative to the tensor , then the Ricci identity takes the form

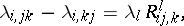where 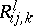is the Riemann curvature tensor determined by the metric tensor 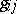of the space 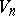(in other words, an alternating second absolute derivative of the tensor field 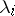in the metric 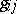is expressed in terms of the Riemann tensor and the components of ).

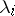For a covariant tensor 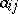of valency 2 the Ricci identity has the form

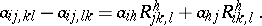In general, for a covariant tensor 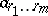of valency 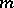the identity has the form

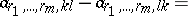Similar identities can be written for contravariant and mixed tensors in 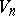. The Ricci identity is used, e.g., in constructions of the geometry of subspaces in 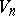as an integrability condition for the principal variational equations from which Gauss' equations and the Peterson–Codazzi equations for subspaces of 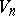are derived.

The identity was established by G. Ricci (see [1]).

References

[1]	G. Ricci, T. Levi-Civita, "Méthodes de calcul différentiel absolu et leurs applications" Math. Ann. , 54 (1901) pp. 125–201
[2]	P.K. [P.K. Rashevskii] Rashewski, "Riemannsche Geometrie und Tensoranalyse" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)
[3]	L.P. Eisenhart, "Riemannian geometry" , Princeton Univ. Press (1949)

Comments

The first Ricci identity is usually called the first Bianchi identity in the West, cf. also Bianchi identity.

References

[a1]	W. Klingenberg, "Riemannian geometry" , de Gruyter (1982) (Translated from German)
[a2]	N.J. Hicks, "Notes on differential geometry" , v. Nostrand (1965)
[a3]	S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1 , Interscience (1963)