Quasi-invariant measure

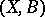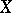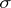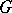A measure on a space that is equivalent to itself under "translations" of this space. More precisely: Let be a measurable space (that is, a set with a distinguished -algebra of subsets of it) and let be a group of automorphisms of it (that is, one-to-one transformations 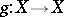that are measurable together with their inverses 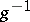with respect to the 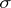-algebra 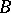). A measure 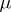on is said to be quasi-invariant (with respect to 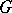) if for any 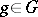the transformed measure 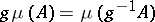, , 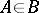is equivalent to the measure 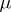(that is, these measures are absolutely continuous with respect to each other, cf. Absolute continuity). If 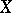is a topological homogeneous space with a continuous locally compact group of automorphisms 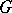(that is, 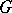acts transitively on 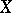and is endowed with a topology such that the mapping 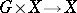, , 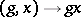is continuous with respect to the product topology on 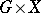) and 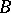is the Borel 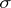-algebra with respect to the topology on , then there exists a quasi-invariant measure that is unique up to equivalence [1]. In particular, a measure on 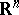is quasi-invariant with respect to all shifts 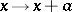, , 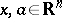if and only if it is equivalent to Lebesgue measure. If the group of transformations is not locally compact, there need not be a quasi-invariant measure; this is the case, for example, in a wide class of infinite-dimensional topological vector spaces [2].

References

[1]	N. Bourbaki, "Elements of mathematics. Integration" , Addison-Wesley (1975) pp. Chapt.6;7;8 (Translated from French)
[2]	I.M. Gel'fand, N.Ya. Vilenkin, "Generalized functions. Applications of harmonic analysis" , 4 , Acad. Press (1964) (Translated from Russian)

Comments

Thus, a quasi-invariant measure is a generalization of a Haar measure on a topological group. On a locally compact group with left Haar measure 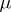a measure is left quasi-invariant (quasi-invariant under left translations) if and only if it is equivalent to .

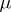There exists no quasi-invariant measure on an infinite-dimensional Hilbert space with respect to the group of all translations (and so, in particular, no Haar measure). Let 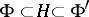be a rigged Hilbert space, with 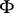a nuclear space with inner product , 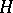the completion of 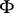, and the dual of 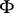. Each 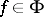defines an element 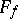in 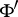, the functional 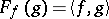. A measure 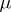on 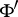is quasi-invariant if 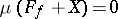for all and with , i.e. if it is quasi-invariant with respect to the group of translations . There exist quasi-invariant measures on such dual spaces of nuclear spaces, [2], Chapt. IV, §5.2.