Point estimator

A statistical estimator whose values are points in the set of values of the quantity to be estimated.

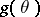Suppose that in the realization of the random vector , taking values in a sample space , , the unknown parameter (or some function ) is to be estimated. Then any statistic producing a mapping of the set into (or into the set of values of ) is called a point estimator of (or of the function to be estimated). Important characteristics of a point estimator 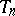are its mathematical expectation

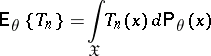and the covariance matrix

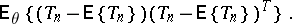The vector is called the error vector of the point estimator 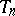. If

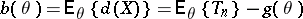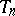is the zero vector for all , then one says that is an unbiased estimator of 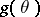or that 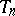is free of systematic errors; otherwise, 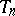is said to be biased, and the vector is called the bias or systematic error of the point estimator. The quality of a point estimator can be defined by means of the risk function (cf. Risk of a statistical procedure).

References

[1]	H. Cramér, "Mathematical methods of statistics" , Princeton Univ. Press (1946)
[2]	I.A. Ibragimov, R.Z. [R.Z. Khas'minskii] Has'minskii, "Statistical estimation: asymptotic theory" , Springer (1981) (Translated from Russian)

References

[a1]	E.L. Lehmann, "Theory of point estimation" , Wiley (1983)