Pendulum equation
An ordinary differential equation of the form
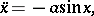 | (*) |
where  is a positive constant. A pendulum equation arises in the study of free oscillations of a mathematical pendulum in a gravity field — a point mass with one degree of freedom attached to the end of a non-extendible and incompressible weightless suspender, the other end of which is fastened on a hinge which permits the pendulum to rotate in a vertical plane. The unknown function
is a positive constant. A pendulum equation arises in the study of free oscillations of a mathematical pendulum in a gravity field — a point mass with one degree of freedom attached to the end of a non-extendible and incompressible weightless suspender, the other end of which is fastened on a hinge which permits the pendulum to rotate in a vertical plane. The unknown function 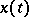 is the deviation angle of the pendulum at time
is the deviation angle of the pendulum at time 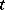 from the lower equilibrium position, measured in radians;
from the lower equilibrium position, measured in radians;
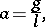 |
where  is the length of the suspender and
is the length of the suspender and 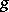 is the gravitational acceleration. The (approximate) equation describing the small oscillations of the pendulum about the lower equilibrium position has the form
is the gravitational acceleration. The (approximate) equation describing the small oscillations of the pendulum about the lower equilibrium position has the form
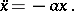 |
The qualitative investigation of the pendulum equation is carried out using the law of conservation of energy, which relates the position and the velocity of the pendulum:
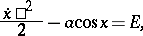 |
where  is the total energy of the pendulum. The time scale can be chosen so that
is the total energy of the pendulum. The time scale can be chosen so that  . Then for an energy value
. Then for an energy value 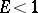 the pendulum performs an oscillatory movement (the velocity changes its sign periodically), whereas for
the pendulum performs an oscillatory movement (the velocity changes its sign periodically), whereas for 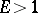 it rotates (the velocity has constant sign). The solution
it rotates (the velocity has constant sign). The solution 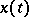 of (*) with initial condition
of (*) with initial condition 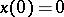 ,
, 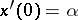 for
for 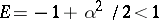 , satisfies the relation
, satisfies the relation
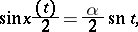 |
where the Jacobi elliptic function 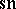 has modulus
has modulus  (cf. Jacobi elliptic functions).
(cf. Jacobi elliptic functions).
Of great practical importance are equations close to the pendulum equation. The presence of a small friction that depends on the position and velocity of the pendulum leads to the equation
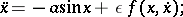 |
the small oscillations of the pendulum with friction are described by the equation
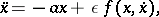 |
a particular case of which is the van der Pol equation. The oscillations of a pendulum for which the length of the suspender varies periodically (the motion of a swing) are described by the Hill equation, an important particular case of which is the Mathieu equation.
References
| [1] | V.I. Arnol'd, "Ordinary differential equations" , M.I.T. (1973) (Translated from Russian) |
| [2] | E. Kamke, "Differentialgleichungen: Lösungen und Lösungsmethoden" , 1. Gewöhnliche Differentialgleichungen , Chelsea, reprint (1971) |
| [3] | A.A. Andronov, A.A. Vitt, A.E. Khaikin, "Theory of oscillators" , Dover, reprint (1987) (Translated from Russian) |
Comments
For  and
and 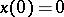 ,
, 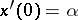 with
with  the motion is periodic with amplitude
the motion is periodic with amplitude
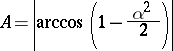 |
and period
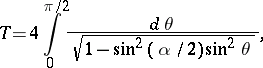 |
which is a complete elliptic integral of the first kind, see [a1]. The periodically-forced damped pendulum
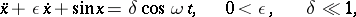 |
and the parametrically-forced damped pendulum
 |
give rise to chaotic solutions. These are analyzed with Melnikov's method in, respectively, [a1] and [a3]. In [a4] the class of problems
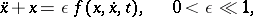 |
is studied with perturbation methods (cf. also Perturbation theory). Special attention is given to the averaging method (cf. e.g. Krylov–Bogolyubov method of averaging).
References
| [a1] | J.K. Hale, "Ordinary differential equations" , Wiley (Interscience) (1969) |
| [a2] | J. Guckenheimer, P. Holmes, "Nonlinear oscillations, dynamical systems, and bifurcation of vectorfields" , Springer (1983) |
| [a3] | S. Wiggins, "Global bifurcations and chaos" , Springer (1988) |
| [a4] | J.A. Sanders, F. Verhulst, "Averaging methods in nonlinear dynamical systems" , Springer (1985) |
| [a5] | V.I. Arnol'd, A. Avez, "Problèmes ergodiques de la mécanique classique" , Gauthier-Villars (1967) (Translated from Russian) |
Pendulum equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pendulum_equation&oldid=48152