Parallelizable manifold

A manifold of dimension admitting a (global) field of frames (cf. also Frame), that is, vector fields that are linearly independent at each point. The field determines an isomorphism of the tangent bundle onto the trivial bundle , which sends a tangent vector to its coordinates with respect to the frame 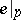and its origin. Therefore a parallelizable manifold can also be defined as a manifold having a trivial tangent bundle. Examples of parallelizable manifolds are open submanifolds of a Euclidean space, all three-dimensional manifolds, the space of an arbitrary Lie group, and the manifolds of frames of an arbitrary manifold. The sphere is a parallelizable manifold only for 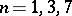. A necessary and sufficient condition for the parallelizability of a 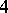-dimensional manifold is the vanishing of the second Stiefel–Whitney characteristic class. In the general case the vanishing of the second characteristic classes of Stiefel— Whitney, Chern and Pontryagin are necessary but not sufficient conditions for a manifold to be parallelizable.

References

[a1]	M. Gromov, "Partial differential relations" , Springer (1986) (Translated from Russian)
[a2]	D. Husemoller, "Fibre bundles" , McGraw-Hill (1966)