Morse inequalities

Inequalities following from Morse theory and relating the number of critical points (cf. Critical point) of a Morse function on a manifold to its homology invariants.

Let 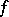be a Morse function on a smooth -dimensional manifold (without boundary) having a finite number of critical points. Then the homology group 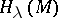is finitely generated and is therefore determined by its rank, , and its torsion rank, (the torsion rank of an Abelian group 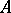with a finite number of generators is the minimal number of cyclic groups in a direct-sum decomposition of which a maximal torsion subgroup of can be imbedded). The Morse inequalities relate the number of critical points of with Morse index to these ranks, and have the form:

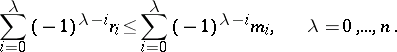For the last Morse inequality is always an equality, so that

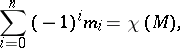where 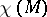is the Euler characteristic of .

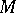The Morse inequalities also hold for Morse functions of a triple 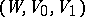, it suffices to replace the groups 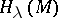by the relative homology groups .

According to the Morse inequalities, a manifold having "large" homology groups does not admit a Morse function with a small number of critical points. It is remarkable that the estimates in the Morse inequalities are sharp: On a closed simply-connected manifold of dimension 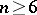there is a Morse function for which the Morse inequalities are equalities (Smale's theorem, see [2]). In particular, on any closed manifold that is homotopically equivalent to the sphere 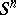, with 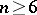, there is a Morse function with two critical points; hence it follows immediately (see Morse theory) that 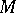is homeomorphic to 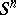(see Poincaré conjecture). A similar application of Smale's theorem allows one to prove theorems on - and -cobordism.

An analogue of the Morse inequalities holds for a Morse function 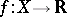on an infinite-dimensional Hilbert manifold, and they relate (for any regular values , , 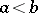of ) the numbers 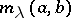of critical points of finite index lying in 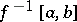, with the rank 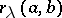and torsion rank of the group , where 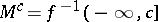. Namely,

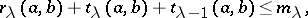For 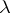large enough the latter inequality becomes an equality.

References

[1]	M. Morse, "The calculus of variations in the large" , Amer. Math. Soc. (1934)
[2]	S. Smale, "Generalized Poincaré's conjecture in dimensions greater than four" Ann. of Math. , 74 (1961) pp. 391–466

Comments

Another version of the Morse inequalities can be stated as follows, cf. [a1].

For a Morse function 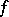one introduces the quantity

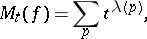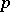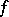where the sum is taken over the critical points of and 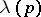is the index of 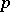relative to . In the compact case this sum is finite, since the critical points are discrete. The polynomial 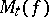, which is also called the Morse polynomial of , has the Poincaré polynomial of the manifold 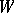as a lower bound in the following sense. Let

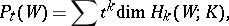where the homology is taken relative to some fixed coefficient field 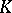. Then the following Morse inequality holds: For every non-degenerate 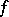there exists a polynomial with non-negative coefficients such that

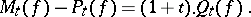References

[a1]	R. Bott, "Lectures on Morse theory, old and new" Bull. Amer. Math. Soc. , 7 : 2 (1982) pp. 331–358
[a2]	J.W. Milnor, "Morse theory" , Princeton Univ. Press (1963)
[a3]	R.S. Palais, "Morse theory on Hilbert manifolds" Topology , 2 (1963) pp. 299–340