Luzin set

projective set

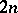A subset of a complete separable metric space, defined by induction as follows. The Luzin sets of class 0 are the Borel sets (cf. Borel set). The Luzin sets of class are continuous images of Luzin sets of class . The Luzin sets of class 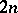are complements of Luzin sets of class 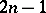. In particular, Luzin sets of class 1, that is, continuous images of Borel sets, are called analytic sets, or 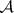-sets or Suslin sets (cf. 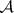-set; Analytic set). The concept of a Luzin set is due to N.N. Luzin [1]. If the sets 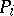are Luzin sets of class , then 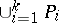and 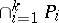are also Luzin sets of class . 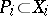If the sets are Luzin sets of class lying in complete separable metric spaces 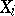, then the direct product (finite or countable) 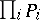is a Luzin set of class in the space . 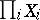A Luzin set of odd class situated in a space coincides with the projection of a set of class 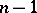situated in . The space of irrational numbers in the interval 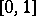contains, for any 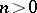, a Luzin set of class that is not a Luzin set of class 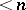; the space also contains sets that are not Luzin sets.

References

[1]	N.N. [N.N. Luzin] Lusin, "Sur un problème de M. Emile Borel et les ensemble projectifs de M. Henri Lebesgue" C.R. Acad. Sci. Paris , 180 (1925) pp. 1318–1320
[2]	N.N. [N.N. Luzin] Lusin, "Leçons sur les ensembles analytiques et leurs applications" , Gauthier-Villars (1930)
[3]	K. Kuratowski, "Topology" , 1 , PWN & Acad. Press (1966) (Translated from French)

Comments

The term "Luzin set" is in the West almost exclusively used for a subset of the real line whose intersection with every nowhere-dense set is countable (see Luzin space). The sets discussed in the main article above are almost exclusively called projective sets (cf. Projective set). The sets of class 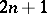are generally called 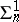-sets and those of class 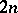are called 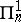-sets. See Descriptive set theory.

All important problems about projective sets have received satisfactory answers during the last three decades, see Descriptive set theory and Luzin problem.

References

[a1]	Y.N. Moschovakis, "Descriptive set theory" , North-Holland (1980)
[a2]	T.J. Jech, "Set theory" , Acad. Press (1978) pp. 523ff (Translated from German)