Korteweg-de Vries equation
KdV-equation
The equation
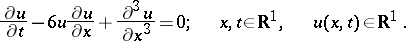 |
It was proposed by D. Korteweg and G. de Vries [1] to describe wave propagation on the surface of shallow water. It can be interpreted using the inverse-scattering method, which is based on presenting the KdV-equation in the form
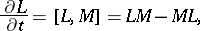 |
where 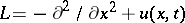 is the one-dimensional Schrödinger operator and
is the one-dimensional Schrödinger operator and
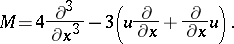 |
The Cauchy problem for the KdV-equation is uniquely solvable in the class of rapidly decreasing functions  with the initial condition:
with the initial condition: 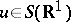 (where
(where 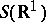 is the Schwartz space). Let
is the Schwartz space). Let
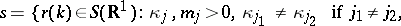 |
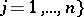 |
be the scattering data for the Schrödinger operator with potential 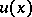 ,
, 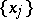 the discrete spectrum,
the discrete spectrum, 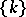 the continuous spectrum and
the continuous spectrum and 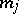 normalization coefficients of the eigen functions. Then
normalization coefficients of the eigen functions. Then
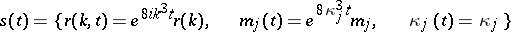 |
are the scattering data for the Schrödinger equation with potential 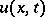 , and the solution
, and the solution 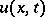 is determined from the scattering data
is determined from the scattering data 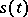 using a certain integral equation. If
using a certain integral equation. If 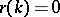 , the latter equation can be solved explicitly; the potentials thus obtained are known as reflection-free, and the corresponding solutions of the KdV-equation are known as
, the latter equation can be solved explicitly; the potentials thus obtained are known as reflection-free, and the corresponding solutions of the KdV-equation are known as  -solitons (see Soliton).
-solitons (see Soliton).
The KdV-equation may be written in Hamiltonian form
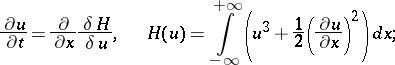 |
here the phase space is 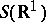 and the Poisson brackets are defined by the bilinear form of the operator
and the Poisson brackets are defined by the bilinear form of the operator 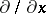 . The mapping
. The mapping  is a canonical transformation to variables of action-angle type. In terms of these new variables the Hamilton equations can be integrated explicitly. The KdV-equation possesses infinitely many integrals of motion:
is a canonical transformation to variables of action-angle type. In terms of these new variables the Hamilton equations can be integrated explicitly. The KdV-equation possesses infinitely many integrals of motion:
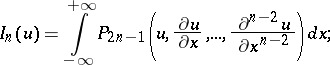 |
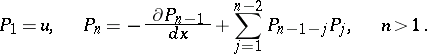 |
All these integrals of motion are integrals in involution, and the Hamiltonian systems that they generate (known as higher Korteweg–de Vries equations) are completely integrable.
Using the integral equations of the inverse problem, one can also find the solution of the Cauchy problem for step-type initial data:
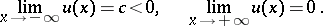 |
As 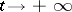 in a neighbourhood of the front, the solution
in a neighbourhood of the front, the solution  decomposes into non-interacting solitons — this is the process of step disintegration.
decomposes into non-interacting solitons — this is the process of step disintegration.
In the case of the Cauchy problem with periodic initial data 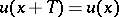 ,
,  , the analogue of reflection-free potentials is provided by potentials for which the Schrödinger operator has finitely many forbidden zones — finite-gap potentials. Periodic and almost-periodic finite-gap potentials are stationary solutions of the higher KdV-equations; the latter constitute completely-integrable finite-dimensional Hamiltonian systems. Any periodic potential can be approximated by a finite-gap potential. Let
, the analogue of reflection-free potentials is provided by potentials for which the Schrödinger operator has finitely many forbidden zones — finite-gap potentials. Periodic and almost-periodic finite-gap potentials are stationary solutions of the higher KdV-equations; the latter constitute completely-integrable finite-dimensional Hamiltonian systems. Any periodic potential can be approximated by a finite-gap potential. Let 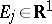 ,
, 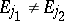 if
if 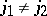 (
(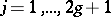 ), be the edges of the bands, and
), be the edges of the bands, and 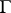 the hyperelliptic curve
the hyperelliptic curve
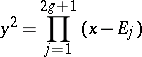 |
over the field 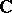 . Then real-valued almost-periodic potentials with the above band edges, as well as solutions of the Cauchy problem, are expressible in terms of
. Then real-valued almost-periodic potentials with the above band edges, as well as solutions of the Cauchy problem, are expressible in terms of 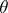 -functions on the Jacobi variety
-functions on the Jacobi variety 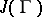 of the curve
of the curve 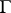 . Subject to certain conditions on the edges, the resulting solutions will be periodic. If one drops the conditions
. Subject to certain conditions on the edges, the resulting solutions will be periodic. If one drops the conditions 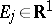 , one obtains complex-valued solutions of the KdV-equation (possibly with poles), which are also called finite-gap potentials.
, one obtains complex-valued solutions of the KdV-equation (possibly with poles), which are also called finite-gap potentials.
References
| [1] | D. Korteweg, G. de Vries, "On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves" Phil Mag. , 39 (1895) pp. 422–443 |
| [2] | C.S. Gardner, J.M. Greene, M.D. [M.D. Krushkal'] Kruskal, R.M. Miura, "Method for solving the Korteweg–de Vries equation" Phys. Rev. Letters , 19 (1967) pp. 1095–1097 |
| [3] | V.E. Zakharov, L.D. Faddeev, "Korteweg–de Vries equation, a completely integrable Hamiltonian system" Funct. Anal. Appl. , 5 (1971) pp. 280–287 Funkt. Anal. Prilozhen. , 5 : 4 (1971) pp. 18–27 |
| [4] | V.A. Marchenko, "Spectral theory of Sturm–Liouville operators" , Kiev (1972) |
| [5] | B.A. Dubrovin, V.B. Matveev, S.P. Novikov, "Nonlinear equations of Korteweg–de Vries type, finite-zone linear operators and Abelian varieties" Russian Math. Surveys , 31 : 1 (1976) pp. 59–146 Uspekhi Mat. Nauk , 31 : 1 (1976) pp. 55–136 |
| [6] | I.A. Kunin, "Theory of elastic media with a microstructure" , Moscow (1975) (In Russian) |
Comments
The Poisson bracket 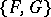 is explicitly given as
is explicitly given as
 |
this being the "bilinear form of the operator / x" referred to above.
In more detail, the scattering data of the Schrödinger operator  consist of: i) a finite number of discrete eigen values
consist of: i) a finite number of discrete eigen values 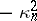 ,
, 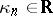 ; ii) normalization coefficients
; ii) normalization coefficients 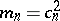 for each element of the discrete spectrum, defined by the requirement that the eigen function
for each element of the discrete spectrum, defined by the requirement that the eigen function 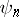 belonging to
belonging to 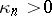 satisfies
satisfies 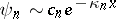 as
as 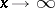 ; and iii) a normalization coefficient for each element of the continuous spectrum
; and iii) a normalization coefficient for each element of the continuous spectrum 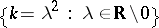 , defined by the requirement that the corresponding eigen functions
, defined by the requirement that the corresponding eigen functions 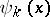 behave like
behave like 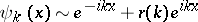 as
as 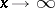 and
and 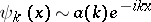 as
as 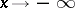 . The coefficient
. The coefficient 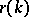 is called a reflection coefficient (and
is called a reflection coefficient (and 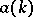 is the corresponding transmission coefficient). (This terminology, as well as the phrase "scattering data" , comes from the "physical picture" where one considers a plane wave coming from
is the corresponding transmission coefficient). (This terminology, as well as the phrase "scattering data" , comes from the "physical picture" where one considers a plane wave coming from 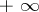 as being scattered by the potential
as being scattered by the potential  ; part of the wave is reflected, part transmitted; and, indeed,
; part of the wave is reflected, part transmitted; and, indeed, 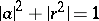 .)
.)
Now if 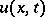 evolves according to the KdV-equation, the spectrum of
evolves according to the KdV-equation, the spectrum of 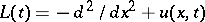 remains constant, i.e. the KdV-equation is an isospectral equation and defines an isospectral flow. This follows readily from the Lax representation
remains constant, i.e. the KdV-equation is an isospectral equation and defines an isospectral flow. This follows readily from the Lax representation 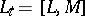 of the KdV-equation.
of the KdV-equation.
The other parts of the spectral data, as  evolves according to the KdV-equation, evolve as indicated above. The (non-linear) mapping which assigns to a potential
evolves according to the KdV-equation, evolve as indicated above. The (non-linear) mapping which assigns to a potential 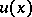 its spectral data is known as the spectral transform. Recovering the potential from its scattering data, by the inverse spectral transform or inverse scattering transform, is done by means of the Gel'fand–Levitan–Marchenko equation (or Gel'fand–Levitan equation):
its spectral data is known as the spectral transform. Recovering the potential from its scattering data, by the inverse spectral transform or inverse scattering transform, is done by means of the Gel'fand–Levitan–Marchenko equation (or Gel'fand–Levitan equation):
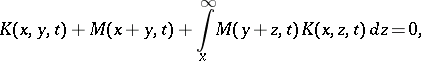 |
where
 |
Then 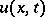 is found by
is found by  . This whole procedure of solving the KdV-equation is known as the inverse spectral-transform method (IST-method, inverse-scattering method), and it can be seen as a non-linear analogue of the Fourier-transform method for solving linear partial differential equations with constant coefficients. In fact, the Fourier transform can be seen as a limit of the spectral transform.
. This whole procedure of solving the KdV-equation is known as the inverse spectral-transform method (IST-method, inverse-scattering method), and it can be seen as a non-linear analogue of the Fourier-transform method for solving linear partial differential equations with constant coefficients. In fact, the Fourier transform can be seen as a limit of the spectral transform.
The modified Korteweg–de Vries equation or mKdV-equation is
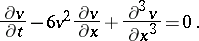 |
It can also be integrated by means of the IST-method, this time using a two-dimensional "L operator" . The two equations are connected by the Miura transformation  . The mKdV-equation is also a member of a hierarchy of completely-integrable equations and there are corresponding Miura transformations between the higher mKdV- and higher KdV-equations.
. The mKdV-equation is also a member of a hierarchy of completely-integrable equations and there are corresponding Miura transformations between the higher mKdV- and higher KdV-equations.
More generally there is a hierarchy of mKdV-like equations associated to each Kac–Moody Lie algebra (cf. Kac–Moody algebra) 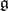 , and then for each simple root of
, and then for each simple root of  there is an associated hierarchy of KdV-equations together with a corresponding Miura transformation, [a5]. These equations are sometimes called Drinfel'd–Sokolov equations. The usual mKdV- and KdV-hierarchies correspond to the Kac–Moody Lie algebra
there is an associated hierarchy of KdV-equations together with a corresponding Miura transformation, [a5]. These equations are sometimes called Drinfel'd–Sokolov equations. The usual mKdV- and KdV-hierarchies correspond to the Kac–Moody Lie algebra 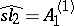 .
.
To the simple Lie algebras one also associates another family of completely-integrable systems: the two-dimensional Toda lattices, also sometimes called Leznov–Saveliev systems. The simplest (associated to 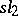 ) is the sine-Gordon equation
) is the sine-Gordon equation
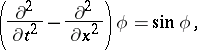 |
or 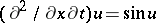 .
.
There is a "duality" between the mKdV-like equations and the corresponding Toda systems, as follows. If 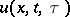 as a function of
as a function of 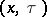 satisfies the Toda lattice equation for
satisfies the Toda lattice equation for 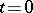 and
and 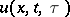 as a function of
as a function of 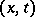 evolves according to the corresponding mKdV-like equation, then
evolves according to the corresponding mKdV-like equation, then 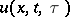 satisfies the Toda lattice equation for all
satisfies the Toda lattice equation for all 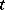 , and vice versa.
, and vice versa.
There is a quantum analogue of the inverse-scattering method, called quantum inverse scattering [a6], [a7]. The (quantum) Yang–Baxter equation plays an important role in this method.
References
| [a1] | M.J. Ablowitz, H. Segur, "Solitons and the inverse scattering transform" , SIAM (1981) |
| [a2] | G.L. Lamb, "Elements of soliton theory" , Wiley (1980) |
| [a3] | A.C. Newell, "Solitons in mathematics and physics" , SIAM (1985) |
| [a4] | F. Caligero, A. Degasperis, "Spectral transform and solitons" , 1 , North-Holland (1982) |
| [a5] | V.G. Drinfel'd, V.V. Sokolov, "Lie algebras and equations of Korteweg–de Vries type" J. Soviet Math. , 30 (1985) pp. 1975–2005 Itogi Nauk. i Tekhn. Sovrem. Probl. Mat. , 24 (1984) pp. 81–180 |
| [a6] | L.A. [L.A. Takhtayan] Takhtajan, "Hamiltonian methods in the theory of solitons" , Springer (1987) (Translated from Russian) |
| [a7] | L.A. [L.A. Takhtayan] Takhtajan, "Integrable models in classical and quantum field theory" , Proc. Internat. Congress Mathematicians (Warszawa, 1983) , PWN & Elsevier (1984) pp. 1331–1346 |
| [a8] | M. Toda, "Nonlinear waves and solitons" , Kluwer (1989) |
| [a9] | V.A. Marchenko, "Nonlinear equations and operator algebras" , Reidel (1988) |
| [a10] | S. Novikov, S.V. Manakov, L.P. Pitaevskii, V.E. Zakharov, "Theory of solitons" , Consultants Bureau (1984) (Translated from Russian) |
Korteweg-de Vries equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Korteweg-de_Vries_equation&oldid=47521