Janet theorem

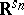In every analytic Riemannian manifold of dimension there exists a neighbourhood of an arbitrarily chosen point having an isometric imbedding into the Euclidean space of dimension 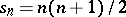. Janet's theorem remains true if 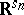is replaced by any analytic Riemannian manifold of dimension with a prescribed point (to which the point chosen in the original manifold must be mapped). Janet's theorem is valid in the case of pseudo-Riemannian manifolds provided that

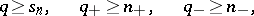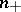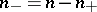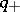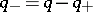where and are the dimensions of the positive and negative parts of the metric tensor on the original manifold, and and are the corresponding dimensions of the target manifold (see [3]). Janet's theorem is the first general imbedding theorem in Riemannian geometry (see Isometric immersion).

Janet's theorem first appeared as a conjecture of L. Schläfli [1], and was proved by M. Janet [2].

References

[1]	L. Schläfli, "Nota alla Memoria del signor Beltrami "Sugli spazi di curvatura costante" " Ann. Mat. Pura. Appl. Ser. 2 , 5 (1873) pp. 178–193
[2]	M. Janet, "Sur la possibilité de plonger un espace Riemannien donné dans un espace euclidien" Ann. Soc. Polon. Math. , 5 (1926) pp. 38–43
[3]	A. Friedman, "Isometric imbedding of Riemannian manifolds into Euclidean spaces" Rev. Modern Physics , 77 (1965) pp. 201–203

Comments

The theorem was also proved by E. Cartan [a1]. A rigorous proof along the lines suggested by Janet was given by C. Burstin [a2]. See also [a3].

References

[a1]	E. Cartan, "Sur la possibilité de plonger un espace riemannien donné dans un espace euclidéen" Ann. Soc. Polon. Math. , 6 (1927) pp. 1–7
[a2]	C. Burstin, Mat. Sb. , 38 (1931) pp. 74–93
[a3]	M. Spivak, "A comprehensive introduction to differential geometry" , 5 , Publish or Perish (1975) pp. 1–5