Jacobi polynomials
Orthogonal polynomials on the interval 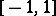 with the weight function
with the weight function
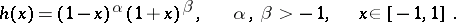 |
The standardized Jacobi polynomials are defined by the Rodrigues formula:
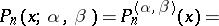 |
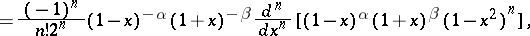 |
and the orthonormal Jacobi polynomials have the form
 |
 |
The polynomial 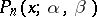 satisfies the differential equation
satisfies the differential equation
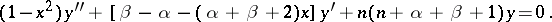 |
When  and
and  , the orthonormal Jacobi polynomials satisfy the following weighted estimate:
, the orthonormal Jacobi polynomials satisfy the following weighted estimate:
 |
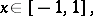 |
where the constant 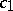 does not depend on
does not depend on  and
and  . At
. At 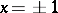 the sequence
the sequence  grows at a rate
grows at a rate  and
and 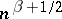 , respectively.
, respectively.
Fourier series in Jacobi polynomials (cf. Fourier series in orthogonal polynomials) inside the interval 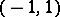 are similar to trigonometric Fourier series. But in neighbourhoods of the end points of this interval, the orthogonality properties of Fourier–Jacobi series are different, because at
are similar to trigonometric Fourier series. But in neighbourhoods of the end points of this interval, the orthogonality properties of Fourier–Jacobi series are different, because at 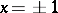 the orthonormal Jacobi polynomials grow unboundedly. The Fourier–Jacobi series of a function
the orthonormal Jacobi polynomials grow unboundedly. The Fourier–Jacobi series of a function 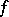 is uniformly convergent on
is uniformly convergent on 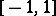 if
if  is
is 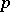 times continuously differentiable on this segment and
times continuously differentiable on this segment and 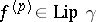 with
with  , where
, where
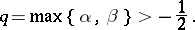 |
Under these conditions the following inequality holds:
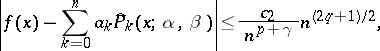 |
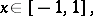 |
where the constant 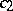 does not depend on
does not depend on  and
and  . On the other hand, when
. On the other hand, when  and
and 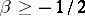 , the remainder in the Fourier–Jacobi series for
, the remainder in the Fourier–Jacobi series for  satisfies the following weighted estimate:
satisfies the following weighted estimate:
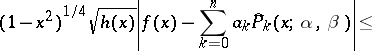 |
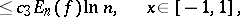 |
where 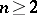 , the constant
, the constant 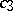 does not depend on
does not depend on  and
and  , and
, and 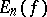 is the best uniform approximation error (cf. Best approximation) of the continuous function
is the best uniform approximation error (cf. Best approximation) of the continuous function 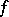 on
on 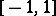 by polynomials of degree not exceeding
by polynomials of degree not exceeding  .
.
The Jacobi polynomials were introduced by C.G.J. Jacobi [1] in connection with the solution of the hypergeometric equation. Special cases of the Jacobi polynomials are: the Legendre polynomials (when 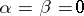 ); the Chebyshev polynomials of the first kind (when
); the Chebyshev polynomials of the first kind (when  ); the Chebyshev polynomials of the second kind (when
); the Chebyshev polynomials of the second kind (when  ); and the ultraspherical polynomials (when
); and the ultraspherical polynomials (when 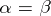 ).
).
See also Classical orthogonal polynomials.
References
| [1] | C.G.J. Jacobi, "Untersuchungen über die Differentialgleichung der hypergeometrischen Reihe" J. Reine Angew. Math. , 56 (1859) pp. 149–165 |
| [2] | P.K. Suetin, "Classical orthogonal polynomials" , Moscow (1978) (In Russian) |
Comments
See also [a4], [a1] and Fourier series in orthogonal polynomials.
Let 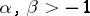 and
and 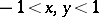 . Then there is a product formula of the form
. Then there is a product formula of the form
 |
with positive measure 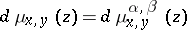 if and only if
if and only if 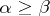 and either
and either  or
or 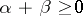 . This yields a positive convolution structure for Jacobi series. For
. This yields a positive convolution structure for Jacobi series. For 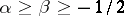 the above measure can be computed explicitly from the addition formula for Jacobi polynomials. See [a1], Lecture 4.
the above measure can be computed explicitly from the addition formula for Jacobi polynomials. See [a1], Lecture 4.
For the dual problem one has
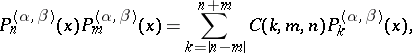 |
with  if
if 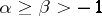 ,
, 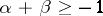 . This yields a positive dual convolution structure for Jacobi series. See [a1], Lecture 5.
. This yields a positive dual convolution structure for Jacobi series. See [a1], Lecture 5.
Jacobi polynomials admit many different group-theoretic interpretations. The three most important ones are as matrix elements of the irreducible representations of 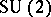 (cf. [a5], Chapt. 3), as
(cf. [a5], Chapt. 3), as  -invariant spherical harmonics on the unit sphere in
-invariant spherical harmonics on the unit sphere in 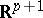 (cf. [a2]) and as zonal spherical functions on the compact symmetric spaces of rank one (cf. [a3], Chapt. 5, §4.3).
(cf. [a2]) and as zonal spherical functions on the compact symmetric spaces of rank one (cf. [a3], Chapt. 5, §4.3).
References
| [a1] | R. Askey, "Orthogonal polynomials and special functions" , Reg. Conf. Ser. Appl. Math. , 21 , SIAM (1975) |
| [a2] | B.L.J. Braaksma, B. Meulenbeld, "Jacobi polynomials as spherical harmonics" Nederl. Akad. Wetensch. Proc. Ser. A , 71 (1968) pp. 384–389 |
| [a3] | S. Helgason, "Groups and geometric analysis" , Acad. Press (1984) pp. Chapt. II, Sect. 4 |
| [a4] | G. Szegö, "Orthogonal polynomials" , Amer. Math. Soc. (1975) |
| [a5] | N.Ya. Vilenkin, "Special functions and the theory of group representations" , Amer. Math. Soc. (1968) (Translated from Russian) |
Jacobi polynomials. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Jacobi_polynomials&oldid=47459