Jackson inequality

An inequality estimating the rate of decrease of the best approximation error of a function by trigonometric or algebraic polynomials in dependence on its differentiability and finite-difference properties. Let be a -periodic continuous function on the real axis, let be the best uniform approximation error of by trigonometric polynomials of degree , i.e.

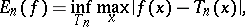and let

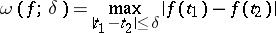be the modulus of continuity of (cf. Continuity, modulus of). It was shown by D. Jackson [1] that

(*)

(where 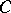is an absolute constant), while if 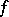has an -th order continuous derivative , , 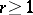then

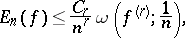where the constant depends on only. S.N. Bernshtein [3] obtained inequality (*) in an independent manner for the case

If 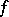is continuous or times continuously differentiable on a closed interval , 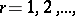and if 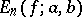is the best uniform approximation error of the functi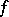on on 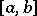by algebraic polynomials of degree , then, for 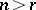one has the relation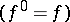

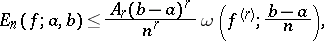where the constant 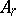depends on only.

The Jackson inequalities are also known as the Jackson theorems or as direct theorems in the theory of approximation of functions. They may be generalized in various directions: to approximation using an integral metric, to approximation by entire functions of finite order, to an estimate concerning the approximation using a modulus of smoothness of order , or to a function of several variables. The exact values of the constants in Jackson's inequalities have been determined in several cases.

References

[1]	D. Jackson, "Ueber die Genauigkeit der Annäherung stetiger Funktionen durch ganze rationale Funktionen gegebenen Grades und trigonometrische Summen gegebener Ordnung" , Göttingen (1911) (Thesis)
[2]	S.M. Nikol'skii, "Approximation of functions of several variables and imbedding theorems" , Springer (1975) (Translated from Russian)
[3]	S.N. Bernshtein, "On the best approximation of continuous functions by polynomials of a given degree (1912)" , Collected works , 1 , Moscow (1952) pp. 11–104
[4]	N.P. Korneichuk, "Extremal problems in approximation theory" , Moscow (1976) (In Russian)
[5]	G.G. Lorentz, "Approximation of functions" , Holt, Rinehart & Winston (1966)

Comments

See also Approximation of functions, direct and inverse theorems.

Let 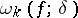be the modulus of continuity of order ,

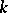Then, more generally,

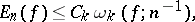where 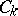is independent of . The best possible coefficients 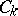were determined by J. Favard. For the interval 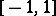the constant 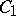is . A result of S.B. Stechkin says that

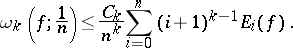References

[a1]	E.W. Cheney, "Introduction to approximation theory" , McGraw-Hill (1966) pp. Chapt. 4
[a2]	G.W. Meinardus, "Approximation von Funktionen und ihre numerische Behandlung" , Springer (1964) pp. Chapt. 1, §5
[a3]	T.J. Rivlin, "An introduction to the approximation of functions" , Dover, reprint (1981)