Hopf ring
A (graded) ring object in the category of (graded) co-commutative co-algebras (cf. Co-algebra). Such an object consists, first, of a sequence  of Abelian group objects in the category. These are better known as commutative Hopf algebras with conjugation. Since they belong to the category, they have a coproduct:
of Abelian group objects in the category. These are better known as commutative Hopf algebras with conjugation. Since they belong to the category, they have a coproduct:
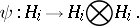 |
Let 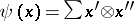 . As group objects in a category of co-algebras, the
. As group objects in a category of co-algebras, the 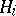 also have a product
also have a product 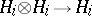 . Below, this product is denoted by
. Below, this product is denoted by  . The "*" product should be thought of as "addition" in the ring as it is the pairing which gives the Abelian group structure. For ring "multiplication" one has
. The "*" product should be thought of as "addition" in the ring as it is the pairing which gives the Abelian group structure. For ring "multiplication" one has
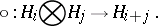 |
As with any ring, there must be a distributive law relating the multiplication and the addition. Chasing diagrams in the category one sees that it is:
 |
Hopf rings arise naturally in the study of the  -spectra associated with generalized cohomology theories. Any generalized cohomology theory,
-spectra associated with generalized cohomology theories. Any generalized cohomology theory, 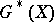 , gives rise to a sequence of spaces,
, gives rise to a sequence of spaces,  , with the property that
, with the property that 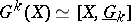 , the homotopy classes of mappings. If
, the homotopy classes of mappings. If 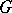 is a multiplicative theory, then
is a multiplicative theory, then 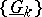 is a graded ring object in the homotopy category. If
is a graded ring object in the homotopy category. If  represents a generalized homology theory and if there is a Künneth isomorphism for the
represents a generalized homology theory and if there is a Künneth isomorphism for the 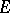 -homology of the spaces in the
-homology of the spaces in the  -spectra for
-spectra for  , then the sequence
, then the sequence  becomes a Hopf ring. One can thus use knowledge of generalized homologies to further the understanding of generalized cohomologies by studying their classifying spaces using Hopf rings.
becomes a Hopf ring. One can thus use knowledge of generalized homologies to further the understanding of generalized cohomologies by studying their classifying spaces using Hopf rings.
There are a number of Hopf rings which have been computed. Examples are  and
and 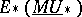 ,
, 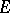 a complex orientable theory, [a9] (the basic reference for Hopf rings);
a complex orientable theory, [a9] (the basic reference for Hopf rings); 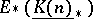 and
and 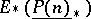 ,
,  a complex orientable theory with
a complex orientable theory with  , [a14] and [a8];
, [a14] and [a8]; 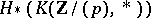 , [a13], § 8;
, [a13], § 8; 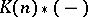 for Eilenberg–MacLane spaces, [a10];
for Eilenberg–MacLane spaces, [a10]; 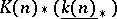 , [a5];
, [a5];  , [a11]; and the breakthrough description of
, [a11]; and the breakthrough description of 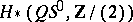 in [a12], and its sequel for
in [a12], and its sequel for  in [a1] followed by corresponding results for odd primes in [a7]. Other references are [a2], [a3], [a4], and [a6].
in [a1] followed by corresponding results for odd primes in [a7]. Other references are [a2], [a3], [a4], and [a6].
Hopf rings have a very rich algebraic structure, useful in two distinct ways: descriptive and computational. All of the above examples have their Hopf rings described with just a few generators and relations. The computations are generally carried out using Hopf ring techniques as well.
References
| [a1] | P.J. Eccles, P.R. Turner, W.S. Wilson, "On the Hopf ring for the sphere" Math. Z. , 224 (2) (1997) pp. 229–233 |
| [a2] | M.J. Hopkins, J.R. Hunton, "The structure of spaces representing a Landweber exact cohomology theory" Topology , 34 (1) (1995) pp. 29–36 |
| [a3] | J.R. Hunton, N. Ray, "A rational approach to Hopf rings" J. Pure Appl. Algebra , 101 (3) (1995) pp. 313–333 |
| [a4] | T. Kashiwabara, "Hopf rings and unstable operations" J. Pure Appl. Algebra , 194 (1994) pp. 183–193 |
| [a5] | R. Kramer, "The periodic Hopf ring of connective Morava 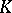 -theory" Ph.D. Thesis, Johns Hopkins Univ. (1990) -theory" Ph.D. Thesis, Johns Hopkins Univ. (1990) |
| [a6] | T. Kashiwabara, N.P. Strickland, P.R. Turner, "Morava 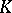 -theory Hopf ring for -theory Hopf ring for 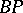 " C. Broto (ed.) etAAsal. (ed.) , Algebraic Topology: New Trends in Localization and Periodicity , Progress in Mathematics , 139 , Birkhäuser (1996) pp. 209–222 " C. Broto (ed.) etAAsal. (ed.) , Algebraic Topology: New Trends in Localization and Periodicity , Progress in Mathematics , 139 , Birkhäuser (1996) pp. 209–222 |
| [a7] | Y. Li, "On the Hopf ring for the sphere" Ph.D. Thesis, Johns Hopkins Univ. (1996) |
| [a8] | D.C. Ravenel, W.S. Wilson, "The Hopf ring for 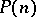 " Canadian J. Math. , 48 (5) (1996) pp. 1044–1063 " Canadian J. Math. , 48 (5) (1996) pp. 1044–1063 |
| [a9] | D.C. Ravenel, W.S. Wilson, "The Hopf ring for complex cobordism" J. Pure Appl. Algebra , 9 (1977) pp. 241–280 |
| [a10] | D.C. Ravenel, W.S. Wilson, "The Morava 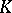 -theories of Eilenberg–Mac Lane spaces and the Conner–Floyd conjecture" Amer. J. Math. , 102 (1980) pp. 691–748 -theories of Eilenberg–Mac Lane spaces and the Conner–Floyd conjecture" Amer. J. Math. , 102 (1980) pp. 691–748 |
| [a11] | N. Strickland, "Bott periodicity and Hopf rings" Ph.D. Thesis, Univ. Manchester (1992) |
| [a12] | P.R. Turner, "Dickson coinvariants and the homology of 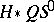 " Math. Z. , 224 (2) (1997) pp. 209–228 " Math. Z. , 224 (2) (1997) pp. 209–228 |
| [a13] | W.S. Wilson, "Brown–Peterson homology: an introduction and sampler" , CBMS , 48 , Amer. Math. Soc. (1982) |
| [a14] | W.S. Wilson, "The Hopf ring for Morava 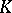 -theory" Publ. RIMS Kyoto Univ. , 20 (1984) pp. 1025–1036 -theory" Publ. RIMS Kyoto Univ. , 20 (1984) pp. 1025–1036 |
Hopf ring. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hopf_ring&oldid=47272