Hoeffding decomposition

Let be independent identically distributed random functions with values in a measurable space (cf. Random variable). For , let

be a measurable symmetric function in variables and consider the -statistics (cf. -statistic)

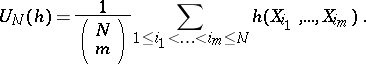The following theorem is called Hoeffding's decomposition theorem, and the representation of the -statistic as in the theorem is called the Hoeffding decomposition of 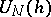(see [a1]):

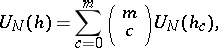where is a symmetric function in arguments and where the -statistics 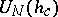are degenerate, pairwise orthogonal in 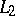(uncorrelated) and satisfy

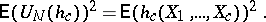The symmetric functions are defined as follows:

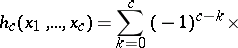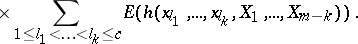Extensions of this decomposition are known for the multi-sample case [a4], under various "uncomplete" summation procedures in the definition of a -statistic, in some dependent situations and for non-identical distributions [a3]. There are also versions of the theorem for symmetric functions that have values in a Banach space.

The decomposition theorem permits one to easily calculate the variance of 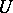-statistics. Since 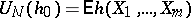and since 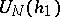is a sum of centred independent identically distributed random variables, the central limit theorem for non-degenerate -statistics is an immediate consequence of the Hoeffding decomposition (cf. also Central limit theorem).

The terminology goes back to [a2].

References

[a1]	M. Denker, "Asymptotic distribution theory in nonparametric statistics" , Advanced Lectures in Mathematics , F. Vieweg (1985)
[a2]	W. Hoeffding, "A class of statistics with asymptotically normal distribution" Ann. Math. Stat. , 19 (1948) pp. 293–325
[a3]	A.J. Lee, "U-statistics. Theory and practice" , Statistics textbooks and monographs , 110 , M. Dekker (1990)
[a4]	E.L. Lehmann, "Consistency and unbiasedness of certain nonparametric tests" Ann. Math. Stat. , 22 (1951) pp. 165–179