H^infinity-control-theory
A branch of control theory where the performance of a dynamical system (cf. Automatic control theory) is appraised in terms of the 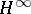 -norm. The Banach space
-norm. The Banach space 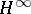 (named after G.H. Hardy, cf. Hardy classes) consists of all complex-valued functions of a complex variable which are analytic and of bounded modulus in the open right half-plane. The norm of such a function is the supremum modulus:
(named after G.H. Hardy, cf. Hardy classes) consists of all complex-valued functions of a complex variable which are analytic and of bounded modulus in the open right half-plane. The norm of such a function is the supremum modulus:
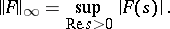 |
By a theorem of Fatou (cf. Fatou theorem), such a function has a boundary value 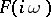 for almost-all
for almost-all 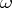 , and, moreover,
, and, moreover,
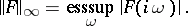 |
The theory of 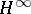 control was initiated by G. Zames [a1], [a2], [a3], who formulated a basic feedback problem as an optimization problem with an operator norm, in particular, an
control was initiated by G. Zames [a1], [a2], [a3], who formulated a basic feedback problem as an optimization problem with an operator norm, in particular, an 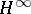 -norm. Relevant contemporaneous works are those of J.W. Helton [a4] and A. Tannenbaum [a5].
-norm. Relevant contemporaneous works are those of J.W. Helton [a4] and A. Tannenbaum [a5].
The theory treats dynamical systems represented as integral operators of the form
 |
Here 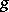 is sufficiently regular to make the input-output mapping
is sufficiently regular to make the input-output mapping 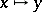 a bounded operator on
a bounded operator on 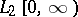 . Taking Laplace transforms gives
. Taking Laplace transforms gives  . The function
. The function 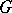 is called the transfer function of the system and it belongs to
is called the transfer function of the system and it belongs to  because the integral operator is bounded. Moreover, the
because the integral operator is bounded. Moreover, the 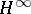 -norm of
-norm of 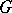 equals the norm of the integral operator, i.e.,
equals the norm of the integral operator, i.e.,
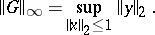 | (a1) |
There are two prototype problems giving rise to an optimality criterion with the 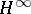 -norm. The first is the problem of robust stability of the feedback system
-norm. The first is the problem of robust stability of the feedback system

Figure: h046020a
Here 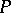 and
and 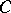 are transfer functions in
are transfer functions in 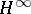 , and
, and 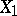 ,
, 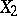 ,
, 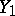 ,
,  are Laplace transforms of signals;
are Laplace transforms of signals;  represents a "plant" , the dynamical system which is to be controlled, and
represents a "plant" , the dynamical system which is to be controlled, and  represents the "controller" (cf. also Automatic control theory). The figure stands for the two equations
represents the "controller" (cf. also Automatic control theory). The figure stands for the two equations
 |
which can be solved to give
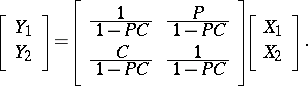 |
Therefore, the input-output mapping for the feedback system has four transfer functions. The feedback system is said to be internally stable if these four transfer functions are all in  . A simple sufficient condition for this is
. A simple sufficient condition for this is 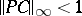 .
.
Internal stability is robust if it is preserved under perturbation of 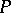 . There are several possible notions of perturbation, typical of which is additive perturbation. So suppose
. There are several possible notions of perturbation, typical of which is additive perturbation. So suppose 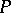 is perturbed to
is perturbed to  , with
, with 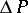 in
in 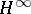 . About
. About 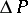 it is assumed that only a bound on
it is assumed that only a bound on 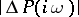 is known, namely,
is known, namely,
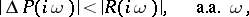 |
where 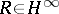 . J.C. Doyle and G. Stein [a6] showed that internal stability is preserved under all such perturbations if and only if
. J.C. Doyle and G. Stein [a6] showed that internal stability is preserved under all such perturbations if and only if
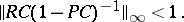 | (a2) |
This leads to the robust stability design problem: Given 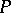 and
and 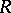 , find
, find 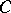 so that the feedback system is internally stable and (a2) holds.
so that the feedback system is internally stable and (a2) holds.
The second problem relates to the same feedback system. Suppose  ,
, 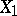 represents a disturbance signal, and the objective is to reduce the effect of
represents a disturbance signal, and the objective is to reduce the effect of 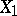 on the output
on the output  . The transfer function from
. The transfer function from 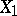 to
to 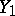 equals
equals 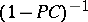 . Suppose, in addition, that the disturbance is not a fixed signal, but can be the output of another system with any input in
. Suppose, in addition, that the disturbance is not a fixed signal, but can be the output of another system with any input in 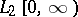 of unit norm; let this latter system have transfer function
of unit norm; let this latter system have transfer function 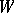 in
in 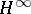 . Then, in view of (a1), the supremal
. Then, in view of (a1), the supremal 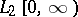 -norm of
-norm of 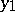 over all such disturbances equals
over all such disturbances equals 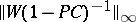 . This leads to the disturbance attenuation problem: Given
. This leads to the disturbance attenuation problem: Given 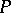 and
and 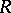 , find
, find 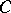 to achieve internal stability and minimize
to achieve internal stability and minimize  .
.
The above two problems are special cases of the more general standard 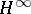 control problem. It can be solved by reduction to the Nehari problem of approximating a function in
control problem. It can be solved by reduction to the Nehari problem of approximating a function in 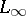 (bounded functions on the imaginary axis) by one in
(bounded functions on the imaginary axis) by one in  . A summary of this theory is in [a7], and a detailed treatment is in [a8].
. A summary of this theory is in [a7], and a detailed treatment is in [a8].
References
| [a1] | G. Zames, "Feedback and complexity, Special plenary lecture addendum" , IEEE Conf. Decision Control , IEEE (1976) |
| [a2] | G. Zames, "Optimal sensitivity and feedback: weighted seminorms, approximate inverses, and plant invariant schemes" , Proc. Allerton Conf. , IEEE (1979) |
| [a3] | G. Zames, "Feedback and optimal sensitivity: model reference transformations, multiplicative seminorms, and approximate inverses" IEEE Trans. Auto. Control , AC-26 (1981) pp. 301–320 |
| [a4] | J.W. Helton, "Operator theory and broadband matching" , Proc. Allerton Conf. , IEEE (1979) |
| [a5] | A. Tannenbaum, "On the blending problem and parameter uncertainty in control theory" Techn. Report Dept. Math. Weizmann Institute (1977) |
| [a6] | J.C. Doyle, G. Stein, "Multivariable feedback design: concepts for a classical modern synthesis" IEEE Trans. Auto. Control , AC-26 (1981) pp. 4–16 |
| [a7] | B.A. Francis, J.C. Doyle, "Linear control theory with an 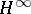 optimality criterion" SIAM J. Control and Opt. , 25 (1987) pp. 815–844 optimality criterion" SIAM J. Control and Opt. , 25 (1987) pp. 815–844 |
| [a8] | B.A. Francis, "A course in 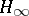 control theory" , Lect. notes in control and inform. science , 88 , Springer (1987) control theory" , Lect. notes in control and inform. science , 88 , Springer (1987) |
H^infinity-control-theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=H%5Einfinity-control-theory&oldid=47156