Grothendieck space

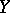A Banach space with the property that for all separable Banach spaces (cf. Separable space), every bounded linear operator 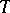from to 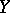is weakly compact (i.e., 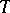sends bounded subsets of into weakly compact subsets of ).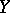

The above property is equivalent to each of the following assertions (see [a4], [a5], [a9]).

1) Every weak- convergent sequence in the dual space of 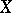is weakly convergent.

2) Every bounded linear operator 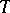from to 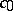is weakly compact.

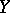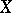3) For all Banach spaces such that has a weak- sequentially compact unit ball, every bounded linear operator from to 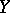is weakly compact.

4) For all weakly compactly generated Banach spaces (i.e., 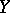is the closed linear span of a relatively weakly compact set), every bounded linear operator from 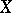to 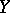is weakly compact.

5) For an arbitrary Banach space 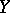, the limit of any weakly convergent sequence of weakly compact operators from to 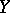is also a weakly compact operator.

6) For any Banach space 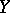, the limit of any strongly convergent sequence of weakly compact operators from 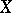to 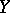is also a weakly compact operator.

Hence, besides the definition given at the beginning, either 1) or 2) can also be used as the definition of a Grothendieck space. Quotient spaces and complemented subspaces of a Grothendieck space are also Grothendieck spaces.

Reflexive Banach spaces are obvious examples of Grothendieck spaces (cf. Reflexive space). Every separable quotient space of a Grothendieck space is necessarily reflexive. The first non-trivial example of a Grothendieck space is the space of continuous functions on a compact Stonean space (i.e., a compact Hausdorff space in which each open set has an open closure) [a6].

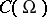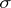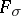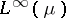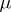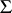Other examples of Grothendieck spaces are: , where is a compact -Stonean space (each open -set has an open closure) or a compact -space (any two disjoint open -sets have disjoint closures) (see [a1], [a10]); , where is a positive measure; , where is a 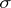-algebra of subsets of ; injective Banach spaces; the Hardy space of all bounded analytic functions on the open unit disc 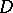[a2]; and von Neumann algebras [a8].

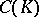A uniformly bounded -semi-group of operators (cf. Semi-group of operators) on a Grothendieck space is strongly ergodic if and only if the weak- closure and the strong closure of the range of the dual operator of the generator coincide [a11]. If is a Grothendieck space, then every sequence of contractions on which converges to the identity in the strong operator topology actually converges in the uniform operator topology (see [a3], [a7]). In particular, this implies equivalence of strong continuity and uniform continuity for contraction 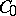-semi-groups on .

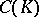References

[a1]	T. Ando, "Convergent sequences of finitely additive measures" Pacific J. Math. , 11 (1961) pp. 395–404
[a2]	J. Bourgain, " is a Grothendieck space" Studia Math. , 75 (1983) pp. 193–216
[a3]	Th. Coulhon, "Suites d'operateurs sur un espace de Grothendieck" C.R. Acad. Sci. Paris , 298 (1984) pp. 13–15
[a4]	J. Diestel, "Grothendieck spaces and vector measures" , Vector and Operator Valued Measures and Applications , Acad. Press (1973) pp. 97–108
[a5]	J. Diestel, J.J. Uhl, Jr., "Vector measures" , Math. Surveys , 15 , Amer. Math. Soc. (1977)
[a6]	A. Grothendieck, "Sur les applications linéaires faiblement compactes d'espaces du type " Canadian J. Math. , 5 (1953) pp. 129–173
[a7]	H.P. Lotz, "Uniform convergence of operators on and similar spaces" Math. Z. , 190 (1985) pp. 207–220
[a8]	H. Pfitzner, "Weak compactness in the dual of a -algebra is determined commutatively" Math. Ann. , 298 (1994) pp. 349–371
[a9]	F. Rábiger, "Beiträge zur Strukturtheorie der Grothendieck-Räume" Sitzungsber. Heidelberger Akad. Wissenschaft. Math.-Naturwiss. Kl. Abh. , 4 (1985)
[a10]	G. L. Seever, "Measures on -spaces" Trans. Amer. Math. Soc. , 133 (1968) pp. 267–280
[a11]	S.-Y. Shaw, "Ergodic theorems for semigroups of operators on a Grothendieck space" Proc. Japan Acad. , 59 (A) (1983) pp. 132–135