Exact endomorphism
of a Lebesgue space 
An endomorphism  of
of  (cf. Metric isomorphism) such that the only measurable decomposition
(cf. Metric isomorphism) such that the only measurable decomposition  that is coarser
that is coarser  than all
than all  , where
, where  is the decomposition into points, is the trivial decomposition with as only element all of
is the decomposition into points, is the trivial decomposition with as only element all of  . An equivalent definition is: There is no measurable decomposition that is invariant (in older terminology — totally invariant) under
. An equivalent definition is: There is no measurable decomposition that is invariant (in older terminology — totally invariant) under  (i.e. is such that
(i.e. is such that 
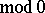 ). Examples of such endomorphisms are a one-sided Bernoulli shift and an expanding mapping.
). Examples of such endomorphisms are a one-sided Bernoulli shift and an expanding mapping.
Exact endomorphisms have strong ergodic properties analogous to those of 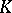 -systems (to which they are related: there is a construction associating an automorphism to some endomorphism — its natural extension; for an exact endomorphism the latter is a
-systems (to which they are related: there is a construction associating an automorphism to some endomorphism — its natural extension; for an exact endomorphism the latter is a  -automorphism). Cf.
-automorphism). Cf.  -system.
-system.
References
| [1] | V.A. Rokhlin, "Exact endomorphisms of a Lebesgue space" Izv. Akad. Nauk SSSR Ser. Mat. , 25 : 4 (1961) pp. 499–530 (In Russian) |
| [2] | I.P. [I.P. Kornfel'd] Cornfel'd, S.V. Fomin, Ya.G. Sinai, "Ergodic theory" , Springer (1982) (Translated from Russian) |
Comments
Instead of "(measurable) decomposition" one also uses (measurable) partition.
The usual definition is as follows: An endomorphism 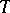 of a Lebesgue space
of a Lebesgue space 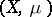 is said to be exact whenever
is said to be exact whenever 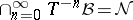 , where
, where 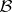 is the given
is the given  -algebra of
-algebra of  and
and 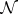 is the
is the 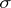 -algebra of subsets of measure 0 or 1. For a proof that expanding mappings are exact with respect to some measure, see e.g. [a1], Sect. III.1.
-algebra of subsets of measure 0 or 1. For a proof that expanding mappings are exact with respect to some measure, see e.g. [a1], Sect. III.1.
References
| [a1] | R. Mañé, "Ergodic theory and differentiable dynamics" , Springer (1987) |
Exact endomorphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Exact_endomorphism&oldid=46864