Equi-affine connection
An affine connection on a smooth manifold  of dimension
of dimension  for which there is a non-zero
for which there is a non-zero  -form
-form 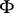 on
on 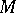 that is covariantly constant with respect to it. The form
that is covariantly constant with respect to it. The form 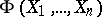 can be interpreted as the volume function of the parallelepiped constructed from the vectors of the fields
can be interpreted as the volume function of the parallelepiped constructed from the vectors of the fields  ; this condition implies the existence of a volume that is preserved by parallel displacement of vectors. If the affine connection on
; this condition implies the existence of a volume that is preserved by parallel displacement of vectors. If the affine connection on 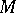 is given by means of a matrix of local connection forms
is given by means of a matrix of local connection forms
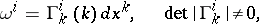 |
 |
and 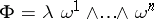 , then the above condition on
, then the above condition on 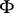 has the form
has the form
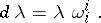 |
Equivalently, an affine connection on 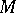 is equi-affine if and only if its holonomy group is the affine unimodular group. In the case of a torsion-free connection this condition is equivalent to the symmetry of the Ricci tensor
is equi-affine if and only if its holonomy group is the affine unimodular group. In the case of a torsion-free connection this condition is equivalent to the symmetry of the Ricci tensor  , that is,
, that is, 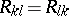 . In the presence of an equi-affine connection the frame bundle of
. In the presence of an equi-affine connection the frame bundle of 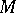 can be reduced to a subbundle with respect to which
can be reduced to a subbundle with respect to which 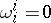 .
.
References
| [1] | A.P. Norden, "Spaces with an affine connection" , Nauka , Moscow-Leningrad (1976) (In Russian) |
Comments
References
| [a1] | J.A. Schouten, "Ricci-calculus. An introduction to tensor analysis and its geometrical applications" , Springer (1954) (Translated from German) |
Equi-affine connection. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Equi-affine_connection&oldid=46839