Endomorphism
of an algebraic system
A mapping of an algebraic system  into itself that is compatible with its structure. Namely, if
into itself that is compatible with its structure. Namely, if  is an algebraic system with a signature consisting of a set
is an algebraic system with a signature consisting of a set  of operation symbols and a set
of operation symbols and a set  of predicate symbols, then an endomorphism
of predicate symbols, then an endomorphism  must satisfy the following conditions:
must satisfy the following conditions:
1)  for any
for any  -ary operation
-ary operation  and any sequence
and any sequence  of elements of
of elements of  ;
;
2)  for any
for any  -place predicate
-place predicate 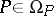 and any sequence
and any sequence  of elements of
of elements of  .
.
The concept of an endomorphism is a special case of that of a homomorphism of two algebraic systems. The endomorphisms of any algebraic system form a monoid under the operation of composition of mappings, whose unit element is the identity mapping of the underlying set of the system (cf. Endomorphism semi-group).
An endomorphism having an inverse is called an automorphism of the algebraic system.
Comments
Thus, by way of one of the simplest examples, an endomorphism of an Abelian group  is a mapping
is a mapping  such that
such that  ,
,  for all elements
for all elements  and
and  in
in  and
and  for all
for all 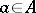 . For an endomorphism
. For an endomorphism  of a ring
of a ring  with a unit 1, the requirements are that
with a unit 1, the requirements are that 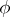 be an endomorphism of the underlying commutative group and that, moreover,
be an endomorphism of the underlying commutative group and that, moreover,  and
and  for all
for all 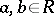 .
.
References
| [a1] | P.M. Cohn, "Universal algebra" , Reidel (1981) |
Endomorphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Endomorphism&oldid=46820