Elliott-Daboussi theorem

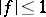The Delange theorem, proved in 1961, gives necessary and sufficient conditions for a multiplicative arithmetic function , of modulus , to possess a non-zero mean value. The unpleasant condition 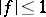was replaced by P.D.T.A. Elliott, in 1975–1980, by boundedness of a semi-norm

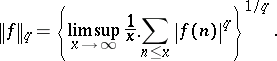More precisely, Elliott showed (see [a4], [a6]) the following result. Assume that 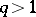and that 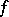is a multiplicative arithmetic function with bounded semi-norm 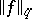. Then the mean value

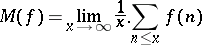of 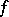exists and is non-zero if and only if

i) the four series

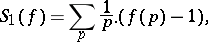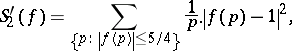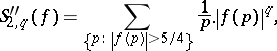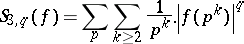are convergent; and

ii) 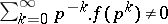for every prime .

H. Daboussi [a3] gave another proof for this result and extended it [a2] to multiplicative functions having at least one non-zero Fourier coefficient 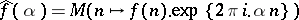; the necessary and sufficient conditions for this to happen are the convergence of the series 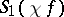, , 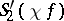, 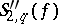and 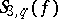for some Dirichlet character .

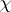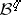See also [a5], [a7], [a8], [a9], [a1]. In fact, the conditions of the Elliott–Daboussi theorem ensure that belongs to the space , which is the 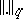-closure of the vector space of linear combinations of the Ramanujan sums 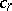, . For details see [a10], Chapts. VI, VII.

References

[a1]	P. Codecà, M. Nair, "On Elliott's theorem on multiplicative functions" , Proc. Amalfi Conf. Analytic Number Theory , 1989 (1992) pp. 17–34
[a2]	H. Daboussi, "Caractérisation des fonctions multiplicatives p.p. 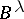à spectre non vide" Ann. Inst. Fourier Grenoble , 30 (1980) pp. 141–166
[a3]	H. Daboussi, "Sur les fonctions multiplicatives ayant une valeur moyenne non nulle" Bull. Soc. Math. France , 109 (1981) pp. 183–205
[a4]	P.D.T.A. Elliott, "A mean-value theorem for multiplicative functions" Proc. London Math. Soc. (3) , 31 (1975) pp. 418–438
[a5]	P.D.T.A. Elliott, "Probabilistic number theory" , I–II , Springer (1979–1980)
[a6]	P.D.T.A. Elliott, "Mean value theorems for functions bounded in mean -power, 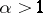" J. Austral. Math. Soc. Ser. A , 29 (1980) pp. 177–205
[a7]	K.-H. Indlekofer, "A mean-value theorem for multiplicative functions" Math. Z. , 172 (1980) pp. 255–271
[a8]	W. Schwarz, J. Spilker, "Eine Bemerkung zur Charakterisierung der fastperiodischen multiplikativen zahlentheoretischen Funktionen mit von Null verschiedenem Mittelwert" Analysis , 3 (1983) pp. 205–216
[a9]	W. Schwarz, J. Spilker, "A variant of proof of Daboussi's theorem on the characterization of multiplicative functions with non-void Fourier–Bohr spectrum" Analysis , 6 (1986) pp. 237–249
[a10]	W. Schwarz, J. Spilker, "Arithmetical functions" , Cambridge Univ. Press (1994)