Discrete distribution
2020 Mathematics Subject Classification: Primary: 60E05 [MSN][ZBL]
A probability distribution (concentrated) on a finite or countably infinite set of points of a sampling space  . More exactly, let
. More exactly, let  be the sample points and let
be the sample points and let
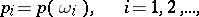 | (1) |
be numbers satisfying the conditions
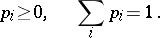 | (2) |
Relations (1) and (2) fully define a discrete distribution on the space  , since the probability measure of any set
, since the probability measure of any set  is defined by the equation
is defined by the equation
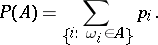 |
Accordingly, the distribution of a random variable  is said to be discrete if it assumes, with probability one, a finite or a countably infinite number of distinct values
is said to be discrete if it assumes, with probability one, a finite or a countably infinite number of distinct values  with probabilities
with probabilities  . In the case of a distribution on the real line, the distribution function
. In the case of a distribution on the real line, the distribution function  has jumps at the points
has jumps at the points 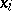 equal to
equal to 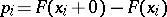 , and is constant in the intervals
, and is constant in the intervals 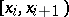 . The following discrete distributions occur most frequently: the binomial distribution, the geometric distribution, the hypergeometric distribution, the negative binomial distribution, the multinomial distribution, and the Poisson distribution.
. The following discrete distributions occur most frequently: the binomial distribution, the geometric distribution, the hypergeometric distribution, the negative binomial distribution, the multinomial distribution, and the Poisson distribution.
Comments
A word of caution. In the Russian literature, 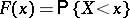 , whereas in Western literature
, whereas in Western literature 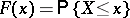 . So the distribution functions are slightly different: left continuous in the Russian literature, and right continuous in the Western literature.
. So the distribution functions are slightly different: left continuous in the Russian literature, and right continuous in the Western literature.
Discrete distribution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Discrete_distribution&oldid=46730