Dirichlet integral

A functional connected with the solution of the Dirichlet problem for the Laplace equation by the variational method. Let be a bounded domain in with boundary of class , let and let the function (cf. Sobolev space). The Dirichlet integral for the function is the expression

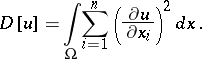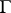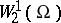For a certain given function on one considers the set of functions from which satisfy the boundary condition 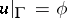. If the set is non-empty, there exists a unique function 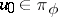for which

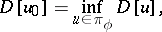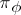and this function is harmonic in . The converse theorem is also true: If a harmonic function belongs to the set , then is attained on it. Thus, is a generalized solution from of the Dirichlet problem for the Laplace equation. However, not for every function 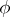it is possible to find a function . There exists even continuous functions on 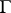for which the set is empty, i.e. the space contains no functions satisfying the condition . The classical solution of the Dirichlet problem for the Laplace equation with such boundary function 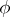cannot have a finite Dirichlet integral and is not a generalized solution from the space .

References

[1]	V.P. Mikhailov, "Partial differential equations" , MIR (1978) (Translated from Russian)

Comments

The restriction of a function (distribution) to a set (in this case the boundary) 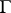is also called the trace of on 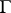in this setting.

See [a1] for a well-known additional reference. Note that the Hilbert space obtained by completion of the set of all -functions with compact support with respect to the scalar product

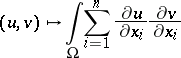can be continuously imbedded into . This observation leads to the introduction of the axiomatic theory of Dirichlet spaces, explaining larger parts of classical potential theory (see, e.g., [a2] or [a3], and Potential theory).

See also Dirichlet principle; Dirichlet variational problem.

References

[a1]	M. Brélot, "Eléments de la théorie classique du potentiel" , Sorbonne Univ. Centre Doc. Univ. , Paris (1959)
[a2]	J. Deny, "Méthodes Hilbertiennes et théorie du potential" M. Brelot (ed.) H. Bauer (ed.) J.-M. Bony (ed.) J. Deny (ed.) G. Mokobodzki (ed.) , Potential theory (CIME, Stresa, 1969) , Cremonese (1970)
[a3]	M. Fukushima, "Dirichlet forms and Markov processes" , North-Holland (1980)