Darboux surfaces

wreath of

Surfaces associated with an infinitesimal deformation of one of them; discovered by G. Darboux [1]. Darboux surfaces form a "wreath" of 12 surfaces, with radius vectors 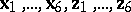satisfying the equations

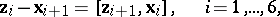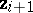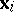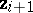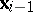where and are in Peterson correspondence, and are in polar correspondence, while 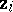and are poles of a 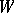-congruence. A similar "wreath" is formed by pairs of isometric surfaces of an elliptic space.

References

[1]	G. Darboux, "Leçons sur la théorie générale des surfaces et ses applications géométriques du calcul infinitésimal" , 4 , Gauthier-Villars (1896)
[2]	V.I. Shulikovskii, "Classical differential geometry in a tensor setting" , Moscow (1963) (In Russian)

Comments

For the notion of a -congruence cf. Congruence of lines.

References

[a1]	G. Fubini, E. Čech, "Introduction á la géométrie projective différentielle des surfaces" , Gauthier-Villars (1931)
[a2]	G. Bol, "Projective Differentialgeometrie" , Vandenhoeck & Ruprecht (1954)