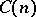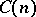Costas array
of order 
An 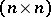 permutation matrix in which the
permutation matrix in which the  line segments connecting pairs of ones in the matrix are distinct as vectors, i.e., no two agree in both magnitude and slope. Thus, if
line segments connecting pairs of ones in the matrix are distinct as vectors, i.e., no two agree in both magnitude and slope. Thus, if  and
and 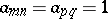 in the matrix, with
in the matrix, with 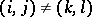 ,
,  , and at least three of these four ordered pairs are distinct, and if
, and at least three of these four ordered pairs are distinct, and if 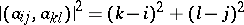 equals
equals 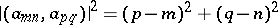 , then
, then
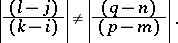 |
All known (1996) general constructions for Costas arrays (see [a3], [a4], [a5]) involve primitive roots in finite fields (cf. Field). The Welch construction, for every prime  , gives a Costas array of order
, gives a Costas array of order 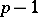 by setting
by setting 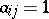 when
when 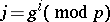 , where
, where 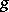 is a primitive root modulo
is a primitive root modulo 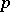 . Removing row
. Removing row 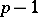 and column
and column 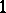 from this construction leaves a Costas array of order
from this construction leaves a Costas array of order 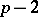 . When
. When 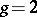 is used as the primitive root modulo
is used as the primitive root modulo  , the array can be further reduced to order
, the array can be further reduced to order 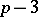 .
.
In Golomb's construction, if 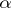 and
and 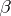 are any two primitive elements in
are any two primitive elements in 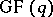 , for
, for 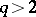 , a Costas array of order
, a Costas array of order 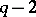 is obtained by setting
is obtained by setting 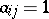 whenever
whenever 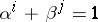 . (The case
. (The case 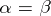 had been discovered by A. Lempel.) If
had been discovered by A. Lempel.) If  , then
, then 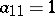 , so that by removing the first row and first column, a Costas array or order
, so that by removing the first row and first column, a Costas array or order 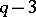 is obtained. As shown in [a6], for every
is obtained. As shown in [a6], for every 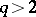 one can find primitive roots
one can find primitive roots 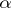 and
and  (not necessarily distinct) with
(not necessarily distinct) with 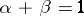 .
.
For 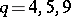 , or any prime
, or any prime  , and for no other finite fields, there is a primitive root
, and for no other finite fields, there is a primitive root  with
with 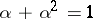 . Thus, in the Lempel construction,
. Thus, in the Lempel construction,  , so that if the first two rows and first two columns are removed, a (symmetric) Costas array or order
, so that if the first two rows and first two columns are removed, a (symmetric) Costas array or order 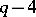 results. For
results. For  , or any prime
, or any prime 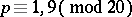 , there are primitive roots
, there are primitive roots 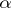 and
and 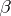 with
with  and
and 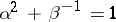 . In this case,
. In this case, 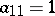 ,
, 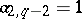 and
and 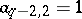 . By successive removal of rows and columns Costas arrays of orders
. By successive removal of rows and columns Costas arrays of orders  ,
, 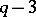 ,
, 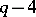 , and
, and 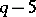 are obtained for these values of
are obtained for these values of  .
.
In some cases a Costas array of order 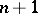 can be obtained from one of order
can be obtained from one of order  by adjoining a
by adjoining a 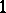 in an exterior corner, i.e., in one of the positions
in an exterior corner, i.e., in one of the positions 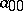 ,
, 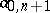 ,
, 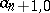 , or
, or  . Several prime-order examples were obtained in this way from a Welch example of order
. Several prime-order examples were obtained in this way from a Welch example of order 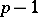 .
.
The total number 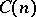 of Costas arrays of order
of Costas arrays of order  is known (1996) for
is known (1996) for 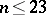 (see the table), with a local maximum at
(see the table), with a local maximum at 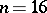 .'
.'
<tbody> </tbody>
|
For a prime number 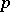 there are
there are  primitive roots, each of which leads to a different Costas array of order
primitive roots, each of which leads to a different Costas array of order 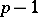 . Since
. Since 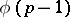 can be arbitrarily large,
can be arbitrarily large, 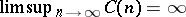 . It has been conjectured, but not proved, that
. It has been conjectured, but not proved, that 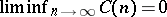 . This would require
. This would require 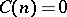 infinitely often. No case of
infinitely often. No case of 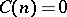 is yet known, but no examples of Costas arrays of orders
is yet known, but no examples of Costas arrays of orders  ,
, 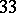 , or
, or 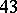 have yet been found. (Many larger orders also lack examples.)
have yet been found. (Many larger orders also lack examples.)
J.P. Costas [a1] first proposed these arrays for an application to frequency hopping sonar signals. Let the  rows represent
rows represent  equally spaced frequencies, and the
equally spaced frequencies, and the  columns
columns  equal duration time intervals. Then the Costas array specifies a permuted order for the
equal duration time intervals. Then the Costas array specifies a permuted order for the  frequencies to be transmitted in
frequencies to be transmitted in  consecutive time intervals. As a sonar (or radar) signal this design has an ideal "thumb-tack" ambiguity function (the two-dimensional auto-correlation function in time and frequency). The horizontal (time) shift measures range (the distance to the target) and the vertical (frequency) shift measures the Doppler (the velocity of the target relative to the observer). For any non-zero shift parallel to the coordinate axes a Costas array has at most one "hit" (coincidence of a
consecutive time intervals. As a sonar (or radar) signal this design has an ideal "thumb-tack" ambiguity function (the two-dimensional auto-correlation function in time and frequency). The horizontal (time) shift measures range (the distance to the target) and the vertical (frequency) shift measures the Doppler (the velocity of the target relative to the observer). For any non-zero shift parallel to the coordinate axes a Costas array has at most one "hit" (coincidence of a 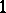 with a
with a 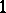 ), and thus gives the least ambiguous reading of the correct range and Doppler in the presence of noise.
), and thus gives the least ambiguous reading of the correct range and Doppler in the presence of noise.
References
| [a1] | J.P. Costas, "Project medior. A medium-oriented approach to sonar signal processing" , HMED Tech. Publ. R66EMH12 , General Electric (1966) |
| [a2] | S.W. Golomb, H. Taylor, "Two-dimensional synchronization patterns with minimum ambiguity" IEEE Trans. Inform. Theory , 28 (1982) pp. 600–604 |
| [a3] | S.W. Golomb, H. Taylor, "Constructions and properties of Costas arrays" Proc. IEEE , 72 (1984) pp. 1143–1163 |
| [a4] | S.W. Golomb, "Algebraic constructions for Costas arrays" J. Combin. Th. A , 37 (1984) pp. 13–21 |
| [a5] | S.W. Golomb, "The 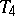 and and 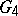 constructions for Costas arrays" IEEE Trans. Inform. Th. , 38 (1992) pp. 1404–1406 constructions for Costas arrays" IEEE Trans. Inform. Th. , 38 (1992) pp. 1404–1406 |
| [a6] | O. Moreno, J. Sotero, "Computational approach to conjecture A of Golomb" Congressus Numerantium , 70 (1990) pp. 7–16 |
| [a7] | J.P. Costas, "A study of a class of detection waveforms having nearly ideal range-doppler ambiguity properties" Proc. IEEE , 72 (1984) pp. 996–1009 |
Costas array. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Costas_array&oldid=46534