Conditional distribution

A function of an elementary event and a Borel set, which for each fixed elementary event is a probability distribution and for each fixed Borel set is a conditional probability.

Let 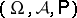be a probability space, 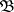the -algebra of Borel sets on the line, 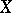a random variable defined on and 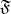a sub--algebra of 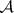. A function 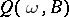defined on 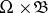is called a (regular) conditional distribution of the random variable 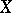with respect to the 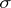-algebra 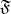if:

a) for fixed the function is 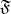-measurable;

b) with probability one, for fixed 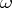the function 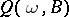is a probability measure on ;

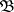c) for arbitrary ,

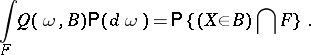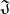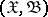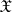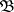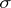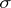Similarly one can define the conditional distribution of a random element with values in an arbitrary measurable space . If is a complete separable metric space and is the -algebra of Borel sets, then the conditional distribution of the random element relative to any -algebra 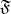, , exists.

The function is called the conditional distribution function of the random variable 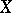with respect to the 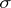-algebra .

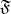The conditional distribution (conditional distribution function) of a random variable with respect to a random variable 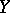is defined as the conditional distribution (conditional distribution function) of 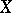with respect to the 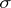-algebra generated by .

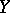The conditional distribution function of a random variable 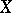with respect to 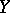is a Borel function of 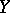; for 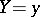its value is called the conditional distribution function of 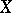for a fixed value o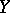f . If 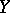has a density 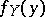, then

where 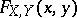is the joint distribution function of 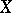and .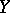

References

[1]	Yu.V. [Yu.V. Prokhorov] Prohorov, Yu.A. Rozanov, "Probability theory, basic concepts. Limit theorems, random processes" , Springer (1969) (Translated from Russian)
[2]	M. Loève, "Probability theory" , Princeton Univ. Press (1963)
[3]	I.I. [I.I. Gikhman] Gihman, A.V. [A.V. Skorokhod] Skorohod, "The theory of stochastic processes" , 1 , Springer (1974) (Translated from Russian)

Comments

Another definition of a conditional distribution is as a function 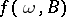of a regular event and a Borel set such that, for fixed , 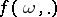is a probability measure and, for fixed 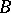, 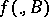is a measurable function.

References

[a1]	L.P. Breiman, "Probability" , Addison-Wesley (1968)