Coadjoint representation
The representation of a Lie group  contragredient to the adjoint representation Ad of
contragredient to the adjoint representation Ad of  (cf. Adjoint representation of a Lie group). The coadjoint representation acts on the dual
(cf. Adjoint representation of a Lie group). The coadjoint representation acts on the dual  of the Lie algebra
of the Lie algebra  of the group
of the group  .
.
If  is a real matrix group, i.e. a subgroup of
is a real matrix group, i.e. a subgroup of  , then
, then  is a subspace of the space
is a subspace of the space  of real matrices of order
of real matrices of order  . Let
. Let 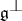 be the orthogonal complement of
be the orthogonal complement of  relative to the bilinear form
relative to the bilinear form
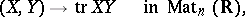 |
let 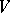 be some subspace of
be some subspace of 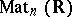 complementary to
complementary to  , and let
, and let 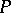 be the projection onto
be the projection onto 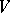 parallel to
parallel to 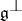 . Then
. Then 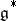 is identified with
is identified with 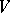 and the coadjoint representation is given by the formula
and the coadjoint representation is given by the formula
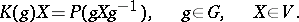 |
The corresponding representation of the Lie algebra  is also called the coadjoint representation. In the case above it is defined by
is also called the coadjoint representation. In the case above it is defined by
 |
The coadjoint representation plays a fundamental role in the orbit method (see [2]). Each  -orbit
-orbit  in the coadjoint representation carries a canonical
in the coadjoint representation carries a canonical  -invariant symplectic structure. In other words, on each orbit
-invariant symplectic structure. In other words, on each orbit  there is a uniquely defined non-degenerate
there is a uniquely defined non-degenerate  -invariant closed differential
-invariant closed differential  -form
-form 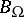 (whence it follows that all
(whence it follows that all 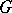 -orbits in the coadjoint representation are even-dimensional). To obtain an explicit expression for
-orbits in the coadjoint representation are even-dimensional). To obtain an explicit expression for  one proceeds as follows. Let
one proceeds as follows. Let  , let
, let  be the orbit through the point
be the orbit through the point 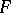 and let
and let 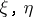 be tangent vectors to
be tangent vectors to  at
at  . There exist
. There exist 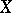 and
and  in
in  such that
such that
 |
Then
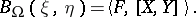 |
For every 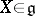 , the vector field
, the vector field  is Hamiltonian with respect to
is Hamiltonian with respect to  ; as its generator (generating function) one can take
; as its generator (generating function) one can take 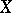 itself, considered as a linear function on
itself, considered as a linear function on 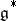 .
.
The stabilizer of a point with orbit of maximal dimension in the coadjoint representation is commutative [1]. The Poisson bracket arising on each orbit generates a single Berezin bracket, which defines the structure of a local Lie algebra (cf. Lie algebra, local), in the space of smooth functions on 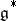 (see [3]). The coordinate expression for the Berezin bracket is
(see [3]). The coordinate expression for the Berezin bracket is
 |
where  are the structure constants of
are the structure constants of  .
.
References
| [1] | P. Bernal, et al., "Représentations des groupes de Lie résolubles" , Dunod (1972) |
| [2] | A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) (Translated from Russian) |
| [3] | A.A. Kirillov, "Local Lie algebras" Russian Math. Surveys , 31 : 4 (1976) pp. 55–75 Uspekhi Mat. Nauk , 31 : 4 (1976) pp. 57–76 |
Coadjoint representation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Coadjoint_representation&oldid=46372