Character of a representation of an associative algebra
A function 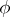 on the associative algebra
on the associative algebra 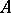 defined by the formula
defined by the formula 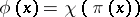 for
for  , where
, where 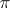 is a representation of
is a representation of  and
and 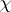 is a linear functional defined on some ideal
is a linear functional defined on some ideal 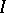 in
in  , satisfying the condition
, satisfying the condition 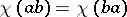 for all
for all 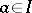 ,
,  . If the representation
. If the representation 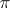 is finite-dimensional or if the algebra
is finite-dimensional or if the algebra  contains a non-zero finite-dimensional operator, then for
contains a non-zero finite-dimensional operator, then for  one usually considers the trace of the operator. Let
one usually considers the trace of the operator. Let 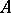 be a
be a  -algebra,
-algebra, 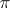 a representation of the
a representation of the  -algebra
-algebra  such that the von Neumann algebra
such that the von Neumann algebra 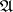 generated by
generated by  is a factor of semi-finite type; let
is a factor of semi-finite type; let  be a faithful normal semi-finite trace on
be a faithful normal semi-finite trace on 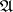 and let
and let  be a linear extension of
be a linear extension of  to an ideal
to an ideal 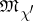 . If the set
. If the set  is non-zero, then the formula
is non-zero, then the formula  ,
,  , determines a character of the representation of the algebra
, determines a character of the representation of the algebra  whose restriction to
whose restriction to  is a character of the
is a character of the  -algebra
-algebra 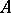 (cf. Character of a
(cf. Character of a 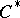 -algebra). In many cases the character of a representation of an algebra determines the representation uniquely, up to a certain equivalence relation; for example, the character of an irreducible finite-dimensional representation determines the representation uniquely up to equivalence; the character of a factor representation of a
-algebra). In many cases the character of a representation of an algebra determines the representation uniquely, up to a certain equivalence relation; for example, the character of an irreducible finite-dimensional representation determines the representation uniquely up to equivalence; the character of a factor representation of a 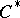 -algebra admitting a trace determines the representation uniquely up to quasi-equivalence.
-algebra admitting a trace determines the representation uniquely up to quasi-equivalence.
References
| [1] | A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) (Translated from Russian) |
| [2] | C.W. Curtis, I. Reiner, "Representation theory of finite groups and associative algebras" , Interscience (1962) |
| [3] | J. Dixmier, "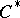 algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
Character of a representation of an associative algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Character_of_a_representation_of_an_associative_algebra&oldid=46314