CA-set
The complement of an  -set in a complete separable metric space
-set in a complete separable metric space  ; that is,
; that is,  is a
is a  -set if
-set if  is an
is an 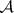 -set, or, in other words a
-set, or, in other words a  -set is a projective set of class 2. There is an example of a
-set is a projective set of class 2. There is an example of a 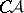 -set that is not an
-set that is not an 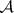 -set. Any
-set. Any  -set is a one-to-one continuous image of some
-set is a one-to-one continuous image of some 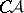 -set (Mazurkiewicz's theorem).
-set (Mazurkiewicz's theorem).
A point 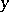 is called a value of order
is called a value of order 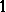 of a mapping
of a mapping 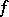 if there is one and only one point such that
if there is one and only one point such that  . The values of order 1 of a
. The values of order 1 of a 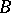 -measurable mapping
-measurable mapping  on an arbitrary Borel set form a
on an arbitrary Borel set form a 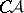 -set (Luzin's theorem). The converse is true: Let
-set (Luzin's theorem). The converse is true: Let 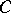 be any
be any  -set belonging to a space
-set belonging to a space  . Then there is a continuous function
. Then there is a continuous function 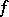 defined on a closed subset of the irrational numbers such that
defined on a closed subset of the irrational numbers such that  is the set of points of order 1 of
is the set of points of order 1 of 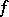 . Kuratowski's reduction theorem: Given an infinite sequence of
. Kuratowski's reduction theorem: Given an infinite sequence of 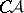 -sets
-sets 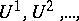 there is a sequence of disjoint
there is a sequence of disjoint  -sets
-sets 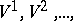 such that
such that 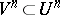 and
and  .
.
References
| [1] | K. Kuratowski, "Topology" , 1 , Acad. Press (1966) (Translated from French) |
Comments
A  -set is also called a co-analytic set, their class is nowadays denoted by
-set is also called a co-analytic set, their class is nowadays denoted by  . See also
. See also 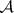 -set.
-set.
CA-set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=CA-set&oldid=46182