Neumann d-bar problem

Neumann DBAR problem, 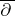-problem, -Neumann problem, DBAR problem, Neumann problem for the Cauchy–Riemann complex

A non-coercive boundary problem for the complex Laplacian. Let be a relatively compact domain of a complex manifold 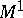of dimension 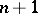with smooth boundary . The Cauchy–Riemann operator 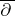(defined on functions on a domain by ) naturally extends to define the Dolbeault complex or Cauchy–Riemann complex

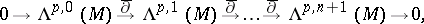where 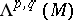is the space of differential forms of type 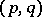on 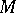. The holomorphic functions are the solutions of 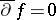and the inhomogeneous equation 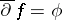(under the necessary compactibility condition 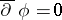) is also of interest. For instance, in connection with the Levi problem: Given 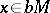, is there a holomorphic function on 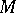which blows up at ? Using a general formalism of D.C. Spencer (and general Hilbert space theory), the problem 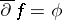leads to the 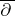-Neumann problem

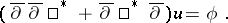(a1)

Here 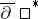is the adjoint of 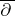, which is defined by 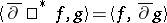, where the inner product is given by integration with respect to the volume form determined by a given Hermitian metric on 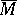. The operator is called the complex Laplacian. If 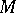is a Kähler manifold, then 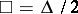, where 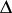is the usual Laplacian of the de Rham complex, cf. de Rham cohomology.

Strictly speaking, equation (a1) should be written as

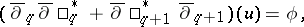(a2)

where 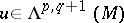, , 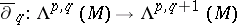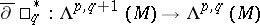; 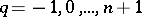, 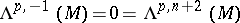. Thus equation (a2) comes naturally equipped with the boundary conditions

(a3)

(a4)

(The -Neumann boundary conditions.) The operator is elliptic, but the boundary conditions are not. Nevertheless, J.J. Kohn was able to prove existence and to provide a systematic analysis of regularity. A main result is the estimate

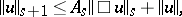where 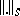are Sobolev norms (cf. Sobolev space). For more details cf. [a1], [a2]. A great deal of additional and related material can be found in [a1]–[a4].

References

[a1]	G.B. Folland, J.J. Kohn, "The Neumann problem for the Cauchy–Riemann complex" , Annals Math. Studies , 75 , Princeton Univ. Press (1972)
[a2]	P.C. Greiner, E.M. Sfein, "Estimates for the 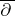-Neumann problem" , Princeton Univ. Press (1977)
[a3]	F. Trèves, "Introduction to pseudodifferential and Fourier integral operators" , 1 , Plenum (1980) pp. Sect. III.8
[a4]	J.J. Kohn, "Methods of partial differential equations in complex analysis" R.O. Wells jr. (ed.) , Several Complex Variables , 1 , Amer. Math. Soc. (1977) pp. 215–240