Rigid analytic space

A variant of the concept of an analytic space related to the case where the ground field is a complete non-Archimedean normed field.

Analytic functions of a -adic variable were considered as long ago as the end of the 19th century in algebraic number theory, whereas the corresponding global object — a rigid analytic space — was introduced by J. Tate only in the early sixties of the 20th century (see [1]). This construction was preceded by a more direct construction on the pattern of the theory of complex-analytic manifolds. The main lack of the latter approach is connected with the fact that the usual local definition of an analytic function as a power-series expansion in a neighbourhood of every point is inconvenient in view of the fact that the ground field is completely disconnected. The analytic functions defined in this way turned out to be "too numerous" (and, correspondingly, the analytic manifolds "too few" ). For example, every compact analytic manifold over is the union of finitely many closed balls (see [2]). Tate's construction starts with the local objects — the affinoid spaces, analogous to the affine varieties in algebraic geometry. Let be the algebra of power series in variables over that converge in the polydisc . The quotient algebras of are called affinoid algebras. These algebras are Noetherian and they have a natural Banach topology in which all ideals are closed and all homomorphisms continuous. It turns out that every maximal ideal of such an algebra has finite codimension, and the space 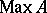of maximal ideals consists, up to conjugacy, of geometric points defined over finite extensions of 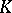. In particular, is the polydisc of unit radius, and, more generally, for arbitrary the space is an analytic subset (cf. Analytic set) of the polydisc. Homomorphisms define morphisms , so that the affinoid spaces form a category.

A rigid structure on a topological space 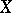is a collection , where is a family of open sets in , called admissible; for each , is a family of coverings of 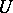by admissible sets (admissible coverings); and 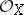is a pre-sheaf of rings on . For an admissible covering it is required that certain natural axioms be satisfied, in particular, admissible coverings are refinable (cf. Refinement), and the pre-sheaf 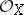must be a sheaf with respect to all admissible coverings by admissible sets. Morphisms of spaces with a rigid structure, and also the concept of the rigid structure induced on a subspace, are defined by analogy with these concepts for ringed spaces. Every affinoid space may be endowed with a canonical rigid structure, which is preserved under morphisms. A rigid analytic space is, by definition, a topological space with a rigid structure on which there exists an admissible covering 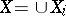such that every , with the induced rigid structure, is isomorphic to an affinoid space equipped with the canonical rigid structure.

Several results have been obtained for rigid analytic spaces that are analogous to known theorems in the theory of complex spaces. Thus, there are analogues of Cartan's Theorems A and B (see Cartan theorem, [4]). More exactly, the coherent sheaves of 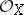-modules on affinoid spaces are uniquely determined by the module of their sections and their cohomology spaces in dimensions . Also valid is the analogue of Grauert's theorem on the coherence of the image of a coherent sheaf under a proper mapping (however, the definition of a proper mapping is very different from the usual one). A 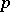-adic analogue of uniformization of algebraic curves and algebraic varieties has been constructed (see ). A connection has been discovered between the concept of a rigid analytic space and that of a formal scheme in algebraic geometry (see ).

References

[1]	J. Tate, "Rigid analytic spaces" Invent. Math. , 12 (1971) pp. 257–289 MR0306196 Zbl 0212.25601
[2]	N.A. Bourbaki, "Variétés différentielles et analytique. Fascicule de résultats" , Eléments de mathématiques , Hermann (1967–1971) pp. Fasc. XXXIII (Par. 1–7); Fasc. XXXVI (Par. 8–15) MR0281115 MR0219078
[3a]	J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) (Translated from French) MR0218496 Zbl 0132.27803
[3b]	J.-P. Serre, "Algèbres de Lie semi-simples complexes" , Benjamin (1966) MR0215886 Zbl 0144.02105
[4]	C. Housel, "Espaces analytiques rigides" , Sem. Bourbaki (1966/67) , Exp. 32 , Benjamin (1968)
[5a]	D. Mumford, "An analytic construction of degenerating curves over complete local rings" Compos. Math. , 24 : 2 (1972) pp. 129–174 MR0352105 Zbl 0243.14010 Zbl 0228.14011
[5b]	D. Mumford, "An analytic construction of degenerating abelian varieties over complete rings" Compos. Math. , 24 : 2 (1972) pp. 239–272 MR0352106 Zbl 0241.14020