Linear ordinary differential equation of the second order
An equation of the form
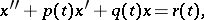 | (1) |
where  is the unknown function and
is the unknown function and 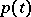 ,
, 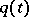 and
and  are given functions, continuous on some interval
are given functions, continuous on some interval 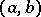 . For any real numbers
. For any real numbers 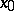 ,
,  , and
, and 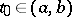 , there is a unique solution
, there is a unique solution 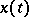 of (1) defined for all
of (1) defined for all 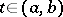 with initial conditions
with initial conditions  ,
, 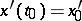 .
.
If 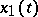 and
and 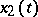 are linearly independent solutions of the corresponding homogeneous equation
are linearly independent solutions of the corresponding homogeneous equation
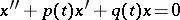 | (2) |
and 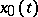 is a particular solution of the inhomogeneous equation (1), then the general solution of (1) is given by the formula
is a particular solution of the inhomogeneous equation (1), then the general solution of (1) is given by the formula
 |
where 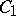 and
and  are arbitrary constants. If one knows one non-zero solution
are arbitrary constants. If one knows one non-zero solution 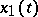 of (2), then a second solution
of (2), then a second solution 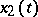 of it, linearly independent of
of it, linearly independent of 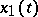 , is given by the formula
, is given by the formula
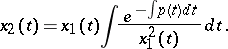 |
If two linearly independent solutions  and
and  of (2) are known, then a particular solution
of (2) are known, then a particular solution 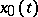 of (1) can be found by the method of variation of constants.
of (1) can be found by the method of variation of constants.
In the study of (2) an important role is played by transformations of it to equations of other types. For example, by the change of variables 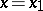 ,
, 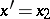 , equation (2) reduces to a normal system of linear equations of the second order; by a change of the unknown function,
, equation (2) reduces to a normal system of linear equations of the second order; by a change of the unknown function,
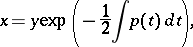 |
equation (2) reduces to the equation 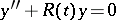 , where
, where
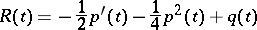 |
is called the invariant of equation (2); by the change of variable 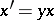 , equation (2) reduces to the Riccati equation
, equation (2) reduces to the Riccati equation
 |
After multiplication by
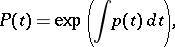 |
equation (2) takes the self-adjoint form
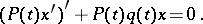 |
Equation (2) can be integrated by quadratures only in isolated cases. The most important special types of non-integrable equations (2) give rise to special functions.
Sturm's theorem on the separation of zeros: If 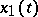 and
and 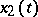 are linearly independent solutions of (2) and
are linearly independent solutions of (2) and 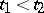 are adjacent zeros of
are adjacent zeros of 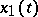 , then on the interval
, then on the interval 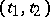 there is exactly one zero of
there is exactly one zero of 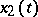 .
.
Suppose that in the equations
 | (3) |
the functions 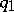 and
and 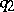 are continuous and
are continuous and 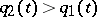 on
on 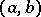 . Then (the comparison theorem): If
. Then (the comparison theorem): If 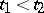 are adjacent zeros of a non-zero solution of the first equation in (3), then on
are adjacent zeros of a non-zero solution of the first equation in (3), then on 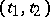 there is at least one zero of any solution of the second equation in (3).
there is at least one zero of any solution of the second equation in (3).
The linear boundary value problem for equation (1) can be stated as follows: Find a solution 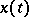 of (1) that satisfies the boundary conditions
of (1) that satisfies the boundary conditions
 |
where  are given constants and
are given constants and
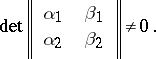 |
The Sturm–Liouville problem for the equation
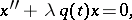 |
where 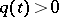 is continuous on
is continuous on 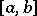 , can be stated as follows: Find those values of the parameter
, can be stated as follows: Find those values of the parameter 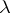 for which this equation has a non-zero solution
for which this equation has a non-zero solution 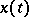 satisfying the boundary conditions
satisfying the boundary conditions 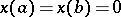 . These values of
. These values of 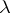 are called the eigen values, and the corresponding solutions are called the eigen functions.
are called the eigen values, and the corresponding solutions are called the eigen functions.
If 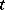 and
and  in equation (2) are complex and the functions
in equation (2) are complex and the functions  and
and 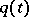 are holomorphic at the point
are holomorphic at the point 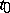 , then for any numbers
, then for any numbers  and
and 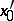 there is a unique complex solution
there is a unique complex solution 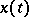 of (2), holomorphic at
of (2), holomorphic at 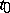 , satisfying the initial conditions
, satisfying the initial conditions 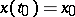 ,
,  . If in the equation
. If in the equation
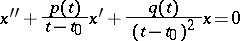 | (4) |
the functions  and
and 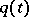 are holomorphic at the point
are holomorphic at the point  and at least one of the numbers
and at least one of the numbers 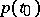 ,
, 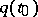 ,
, 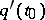 is non-zero, then
is non-zero, then 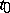 is called a regular singular point for equation (4). In a neighbourhood of such a point one looks for a solution of (4) in the form of a generalized power series
is called a regular singular point for equation (4). In a neighbourhood of such a point one looks for a solution of (4) in the form of a generalized power series
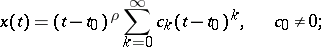 | (5) |
here 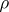 is found from the defining equation
is found from the defining equation
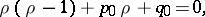 |
where 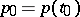 and
and 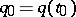 . Suppose that the roots
. Suppose that the roots 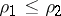 of this equation are real. If
of this equation are real. If  is not an integer, then there are two linearly independent solutions of the form (5), for
is not an integer, then there are two linearly independent solutions of the form (5), for  and for
and for 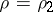 . If
. If 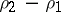 is an integer, then, generally speaking, there is only one solution of the form (5), for
is an integer, then, generally speaking, there is only one solution of the form (5), for  ; the second solution has a more complicated form (see [3]–[6]).
; the second solution has a more complicated form (see [3]–[6]).
References
| [1] | L.S. Pontryagin, "Ordinary differential equations" , Addison-Wesley (1962) (Translated from Russian) |
| [2] | E. Kamke, "Differentialgleichungen: Lösungen und Lösungsmethoden" , 1. Gewöhnliche Differentialgleichungen , Chelsea, reprint (1947) |
| [3] | G. Sansone, "Ordinary differential equations" , 1–2 , Zanichelli (1948–1949) (In Italian) |
| [4] | P. Hartman, "Ordinary differential equations" , Birkhäuser (1982) |
| [5] | F. Tricomi, "Repertorium der Theorie der Differentialgleichungen" , Springer (1968) |
| [6] | V.V. Golubev, "Vorlesungen über Differentialgleichungen im Komplexen" , Deutsch. Verlag Wissenschaft. (1958) (Translated from Russian) |
Comments
See also Differential equation, ordinary; Linear ordinary differential equation.
References
| [a1] | E.L. Ince, "Ordinary differential equations" , Dover, reprint (1956) |
| [a2] | E.A. Coddington, N. Levinson, "Theory of ordinary differential equations" , McGraw-Hill (1955) pp. Chapts. 13–17 |
Linear ordinary differential equation of the second order. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Linear_ordinary_differential_equation_of_the_second_order&oldid=43413