Chinese remainder theorem

Let be a commutative ring with identity and let be a collection of ideals in such that for any . Then, given any set of elements , there exists an such that , . In the particular case when 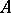is the ring of integers 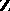, the Chinese remainder theorem states that for any set of pairwise coprime numbers there is an integer giving pre-assigned remainders on division by . In this form the Chinese remainder theorem was known in ancient China; whence the name of the theorem.

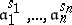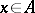The most frequent application of the Chinese remainder theorem is in the case when is a Dedekind ring and , where the are distinct prime ideals in . (If satisfy the condition of the theorem, then so do for any natural numbers .) In this case, the Chinese remainder theorem implies that for any set there exists an such that the decomposition of the principal ideal 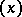into a product of prime ideals has the form

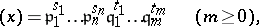where the ideals are pairwise distinct (the theorem on the independence of exponents).

References

[1]	A.I. Kostrikin, "Introduction to algebra" , Springer (1982) (Translated from Russian)
[2]	S. Lang, "Algebra" , Addison-Wesley (1974)
[3]	S. Lang, "Algebraic numbers" , Addison-Wesley (1964)