Order topology

The topology 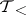on a linearly ordered set 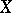, with linear order , which has a base consisting of all possible intervals of .

Comments

Here "interval" is used in the sense of "open interval" , i.e. a set of the form

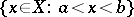where 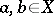(or possibly 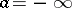and/or 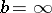). The order topology may be considered on partially ordered as well as linearly ordered sets; on a linearly ordered set it coincides with the interval topology which has the closed intervals

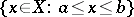as a subbase for the closed sets, but in general it is different. On a complete linearly ordered set, the order topology is characterized by order convergence: that is, a net (see Generalized sequence) 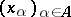converges to a point if and only if there are an increasing net 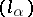and a decreasing net 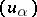, indexed by the same directed set 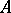, such that 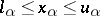for all 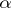and .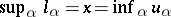

References

[a1]	G. Birkhoff, "Lattice theory" , Colloq. Publ. , 25 , Amer. Math. Soc. (1973)
[a2]	O. Frink, "Topology in lattices" Trans. Amer. Math. Soc. , 51 (1942) pp. 569–582
[a3]	A.J. Ward, "On relations between certain intrinsic topologies in partially ordered sets" Proc. Cambridge Philos. Soc. , 51 (1955) pp. 254–261