Reproducing-kernel Hilbert space

Let be a Hilbert space of functions defined on an abstract set .

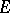Let 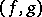denote the inner product and let 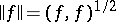be the norm in 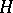. The space is called a reproducing-kernel Hilbert space if there exists a function 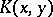, the reproducing kernel, on 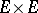such that:

1) 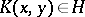for any ;

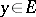2) for all 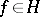(the reproducing property). From this definition it follows that the value at a point 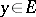is a continuous linear functional in :

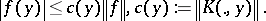The converse is also true. The following theorem holds: A Hilbert space of functions on a set is a reproducing-kernel Hilbert space if and only if 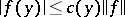for all .

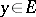By the Riesz theorem, the above assumption implies the existence of a linear functional 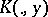such that 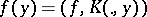. By the construction, the kernel 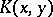is the reproducing kernel for .

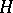An example of a construction of a reproducing-kernel Hilbert space is the rigged triple of Hilbert spaces 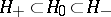, which is defined as follows [a5] (cf. also Rigged Hilbert space). Let 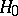be a Hilbert space of functions, let be a linear densely defined self-adjoint operator on 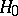, 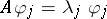(the eigenvalues 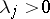are counted according to their multiplicities) and assume that

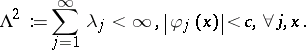Define to be the Hilbert space with inner product 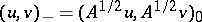. 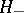is the completion of 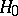in the norm 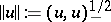. Let 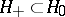be the dual space to 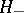with respect to . Then the inner product in 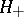is defined by the formula 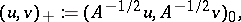and 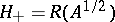, equipped with the inner product 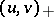, is a Hilbert space.

Define 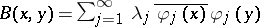, where the overline stands for complex conjugation. For any 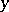, one has 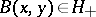. Indeed,

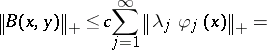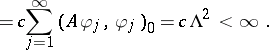Furthermore,

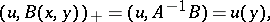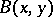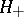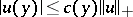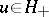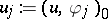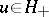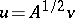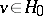
so that is the reproducing kernel in . Moreover , where is a constant independent of . Indeed, if and , then , , 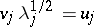and .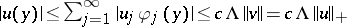

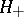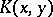Thus is a reproducing kernel Hilbert space with the reproducing kernel defined above. If is a function on 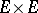such that

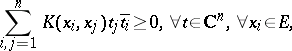(a1)

then one can define a pre-Hilbert space 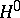of functions of the form

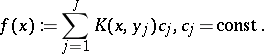The inner product of two functions from 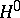is defined by

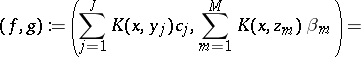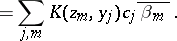This definition makes sense because of (a1) and because of reproducing property 2). In particular, 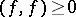, as follows from (a1), and if 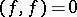then , as follows from property 2).

Indeed,

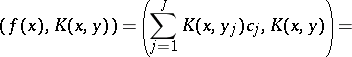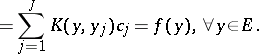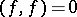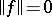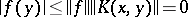Thus, if , then and , so 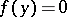as claimed.

Denote by 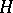the completion of 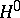in the norm 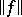. Then is a reproducing-kernel Hilbert space and 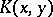is its reproducing kernel.

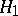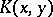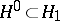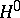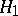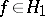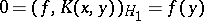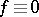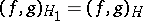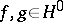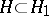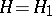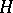A reproducing-kernel Hilbert space is uniquely defined by its reproducing kernel. Indeed, if is another reproducing-kernel Hilbert space with the same reproducing kernel , then and is dense in : If , for all , then . Using this and the equality for all , one can check that and vice versa, so , that is, and consist of the same set of elements. Moreover, the norms in and 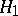are equal. Indeed, take an arbitrary 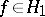and a sequence 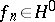, 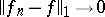. Then

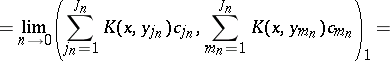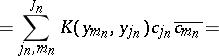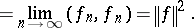Thus, the norms in 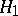and 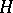are equal, as claimed, and so are the inner products (by the polarization identity).

Define a linear operator 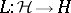, , 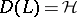where 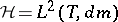and is the range of 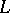, which will be equipped with the structure of a Hilbert space below:

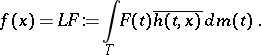(a2)

Here, 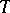is a domain in 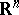and 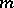is a positive measure on 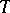, , 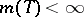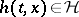for all 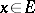, and it is assumed that is injective, that is, the system 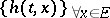is total in 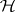(cf. also Total set).

Define

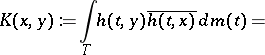(a3)

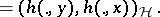This kernel clearly satisfies condition (a1) and therefore is a reproducing kernel for the reproducing-kernel Hilbert space 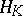which it generates. Clearly 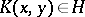for all 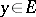. If 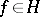, that is, 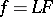, , 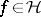then

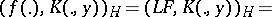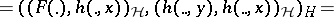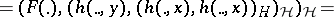if one equips 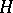with the inner product such that 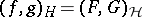. This requirement is formally equivalent to the following one: 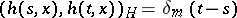, where 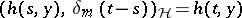, so that the distributional kernel 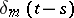is not the usual delta-function, but the one which acts by the rule

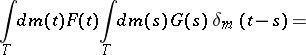and formally one has .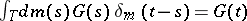

With the inner product 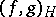, the linear set 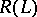becomes a Hilbert space:

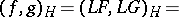(a4)

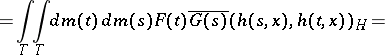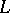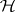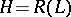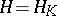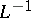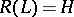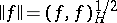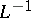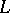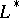Thus, this inner product makes an isometric operator defined on all of and makes a (complete) Hilbert space, namely , a reproducing-kernel Hilbert space. Since is assumed injective, it follows that is defined on all of and, since is complete in the norm , one concludes that is continuous (by the Banach theorem). Consequently, is a co-isometry, that is, , where is the adjoint operator to 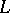. If 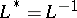, then one can write an inversion formula for the linear transform 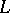similar to the well-known inversion formula for the Fourier transform. Formally one has:

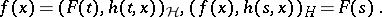The space 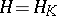is the reproducing-kernel Hilbert space generated by kernel (a3) which is the reproducing kernel for 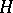. The above formal inversion formulas may be of practical interest if the norm in is a standard one. In this case the second formula should be suitably interpreted, since 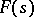is defined at 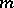-almost all .

In [a6] it is claimed that the characterization of the range of the linear operator , defined in (a3), can be given as follows: 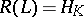, where is the reproducing-kernel Hilbert space generated by kernel (a3).

However, in fact such a characterization does not give, in general, practically useful necessary and sufficient conditions for because the norm in is not defined in terms of standard norms such as Sobolev or Hölder ones (see [a3], [a4], [a5]). However, when the norm in 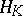is equivalent to a standard norm, the above characterization becomes efficient (see [a3], [a4], [a5], and also [a6]).

Many concrete examples of reproducing-kernel Hilbert spaces can be found in [a1], [a2] and [a6].

The papers [a1] and [a7] are important in this area, the book [a6] contains many references, while [a2] is an earlier book important for the development of the theory of reproducing-kernel Hilbert spaces.

References

[a1]	N. Aronszajn, "Theory of reproducing kernels" Trans. Amer. Math. Soc. , 68 (1950) pp. 337–404
[a2]	S. Bergman, "The kernel function and conformal mapping" , Amer. Math. Soc. (1950)
[a3]	A.G. Ramm, "On the theory of reproducing kernel Hilbert spaces" J. Inverse Ill-Posed Probl. , 6 : 5 (1998) pp. 515–520
[a4]	A.G. Ramm, "On Saitoh's characterization of the range of linear transforms" A.G. Ramm (ed.) , Inverse Problems, Tomography and Image Processing , Plenum (1998) pp. 125–128
[a5]	A.G. Ramm, "Random fields estimation theory" , Longman/Wiley (1990)
[a6]	S. Saitoh, "Integral transforms, reproducing kernels and their applications" , Pitman Res. Notes , Longman (1997)
[a7]	L. Schwartz, "Sous-espaces hilbertiens d'espaces vectoriels topologiques et noyaux associès (noyaux reproduisants)" J. Anal. Math. , 13 (1964) pp. 115–256