Hahn-Banach theorem
Let 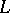 be a linear manifold in a real or complex vector space
be a linear manifold in a real or complex vector space 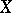 . Suppose
. Suppose 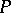 is a semi-norm on
is a semi-norm on  and suppose
and suppose 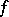 is a linear functional defined on
is a linear functional defined on 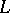 which satisfies
which satisfies
 | (*) |
for every  . Then
. Then 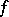 can be extended to a linear functional
can be extended to a linear functional 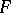 on all of
on all of 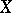 such that
such that
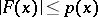 |
for all 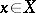 . Such is an extension is not uniquely determined.
. Such is an extension is not uniquely determined.
In the case of a real space 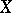 the semi-norm can be replaced by a positively-homogeneous subadditive function, and the inequality (*) by the one-sided inequality
the semi-norm can be replaced by a positively-homogeneous subadditive function, and the inequality (*) by the one-sided inequality  , which remains valid for the extended functional. If
, which remains valid for the extended functional. If 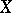 is a Banach space, then for
is a Banach space, then for 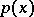 one can take
one can take 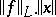 , and then
, and then 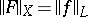 . The theorem was proved by H. Hahn (1927), and independently by S. Banach (1929).
. The theorem was proved by H. Hahn (1927), and independently by S. Banach (1929).
References
| [1] | H. Hahn, "Ueber lineare Gleichungsysteme in linearen Räume" J. Reine Angew. Math. , 157 (1927) pp. 214–229 |
| [2a] | S. Banach, "Sur les fonctionelles linéaires" Studia Math. , 1 (1929) pp. 211–216 |
| [2b] | S. Banach, "Sur les fonctionelles linéaires II" Studia Math. , 1 (1929) pp. 223–239 |
| [3] | A.N. Kolmogorov, S.V. Fomin, "Elements of the theory of functions and functional analysis" , 1–2 , Graylock (1957–1961) (Translated from Russian) |
| [4] | L.V. Kantorovich, G.P. Akilov, "Functional analysis" , Pergamon (1982) (Translated from Russian) |
Comments
A real-valued function 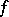 is called subadditive if
is called subadditive if 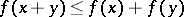 for all
for all 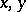 in its domain such that
in its domain such that 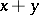 lies in its domain.
lies in its domain.
References
| [a1] | N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Interscience (1958) |
| [a2] | G. Köthe, "Topological vector spaces" , 1 , Springer (1969) |
Hahn-Banach theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hahn-Banach_theorem&oldid=35765