Dirichlet convolution

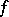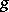The Dirichlet convolution of two arithmetical functions and is defined as

where the sum is over the positive divisors 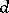of (cf. also Arithmetic function). General background material on the Dirichlet convolution can be found in, e.g., [a1], [a6], [a8].

Sums of the form played an important role from the very beginning of the theory of arithmetical functions. Many results from early times involved these sums. For example, in 1857 J. Liouville published a long list of arithmetical identities of this type (see [a5]). It is fruitful to treat the sums 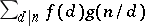as giving a binary operation on the set of arithmetical functions (cf. also Binary relation). This aspect was introduced by E.T. Bell [a2] and M. Cipolla [a3] in 1915.

The set of arithmetical functions forms a commutative ring with unity under the usual addition and the Dirichlet convolution. An arithmetical function possesses a Dirichlet inverse if and only if 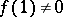. For example, the Dirichlet inverse of the constant function 1 is the Möbius function 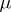. The Möbius inversion formula states that

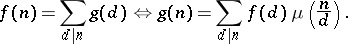The relation of the Dirichlet convolution with Dirichlet series is also important.

There are many analogues and generalizations of the Dirichlet convolution; for example, E. Cohen [a4] defined the unitary convolution as

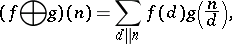where the sum is over the positive divisors of such that 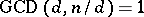, see also [a10]. W. Narkiewicz [a7] developed a more general convolution:

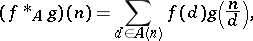where, for each , 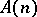is a subset of the set of the positive divisors of . See [a9] for a survey of various binary operations on the set of arithmetical functions.

References

[a1]	T.M. Apostol, "Introduction to analytic number theory" , Springer (1976)
[a2]	E.T. Bell, "An arithmetical theory of certain numerical functions" Univ. Wash. Publ. Math. Phys. Sci. , I : 1 (1915)
[a3]	M. Cipolla, "Sui principi del calculo arithmetico integrale" Atti Accad. Gioenia Cantonia , 5 : 8 (1915)
[a4]	E. Cohen, "Arithmetical functions associated with the unitary divisors of an integer" Math. Z. , 74 (1960) pp. 66–80
[a5]	L.E. Dickson, "History of the theory of numbers" , I , Chelsea, reprint (1952)
[a6]	P.J. McCarthy, "Introduction to arithmetical functions" , Springer (1986)
[a7]	W. Narkiewicz, "On a class of arithmetical convolutions" Colloq. Math. , 10 (1963) pp. 81–94
[a8]	R. Sivaramakrishnan, "Classical theory of arithmetic functions" , Monographs and Textbooks in Pure and Applied Math. , 126 , M. Dekker (1989)
[a9]	M.V. Subbarao, "On some arithmetic convolutions" , The Theory of Arithmetic Functions , Lecture Notes in Mathematics , 251 , Springer (1972) pp. 247–271
[a10]	R. Vaidyanathaswamy, "The theory of multiplicative arithmetic functions" Trans. Amer. Math. Soc. , 33 (1931) pp. 579–662