Translation-invariant metric

invariant metric

A metric 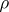on a vector or linear space 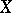such that 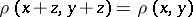for all 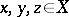. A norm or an 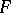-norm, (cf. (the editional comments to) Universal space for a definition of this notion), defines a translation-invariant metric 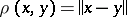. If 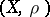is a metric linear space, i.e. a vector space with a metric such that addition and scalar multiplication are continuous, then there is an invariant metric 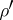on 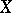that is equivalent to the original one, [a2]. Two metrics 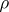, 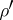on 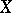are equivalent if they induce the same topology.

References

[a1]	S. Rolewicz, "Metric linear spaces" , Reidel (1987) pp. §1.1
[a2]	S. Kakutani, "Über die Metrisation der topologischen Gruppen" Proc. Imp. Acad. Tokyo , 12 (1936) pp. 82–84