Mal'tsev local theorems

Theorems on transferring properties of local parts of a model (in logic) to the whole model, established by A.I. Mal'tsev. A system 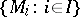of subsets of a set is called a local covering of it if each element of the set is contained in some 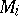and any two 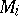, 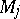are contained in a third subset 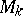. Examples of local coverings are: the system of all finite subsets of a set, and the system of all finitely-generated subgroups of a given group. A model 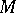locally has a property 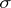if there is a local covering of 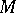consisting of submodels with the property 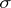. 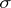A local theorem holds for a property (and a corresponding class of models) if every model locally having property has the property in the large.

A source of a great variety of local theorems is the following fundamental local theorem of Mal'tsev (or the compactness theorem of the restricted predicate calculus) [1]: If each finite subsystem of an infinite system of axioms of the restricted predicate calculus is consistent, then the whole system is consistent. Mal'tsev [2] gave a general method for obtaining concrete local theorems in group theory with the help of the fundamental local theorem, thus making a major contribution to model theory. Later, by improving the method itself, he proved [3] a local theorem for any property described by so-called quasi-universal axioms. The question of the validity of a local theorem for a property , which had previously been investigated separately for each 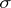, has thus been reduced to a common and quite "grammatical" question: Is it possible to describe by universal axioms?

References

[1]	A.I. Mal'tsev, "Investigation in the realm of mathematical logic" Mat. Sb. , 1 : 3 (1936) pp. 323–336 (In Russian)
[2]	A.I. Mal'tsev, "A general method for obtainging local theorems in group theory" Uchen. Zap. Ivanovsk. Gos. Ped. Inst. , 1 : 1 (1941) pp. 3–9 (In Russian)
[3]	A.I. Mal'tsev, "Model correspondences" Izv. Akad. Nauk. SSSR Ser. Mat. , 23 : 3 (1959) pp. 313–336 (In Russian)
[4]	M.I. Kargapolov, J.I. [Yu.I. Merzlyakov] Merzljakov, "Fundamentals of the theory of groups" , Springer (1979) (Translated from Russian)

Comments

English translations of references [1], [2] and [3] may be found as Chapts. 1, 2 and 11 in [a1].

References

[a1]	A.I. [A.I. Mal'tsev] Mal'cev, "The metamathematics of algebraic systems, Collected papers 1936–1967" , North-Holland (1971) (Translated from Russian)
[a2]	C.C. Chang, H.J. Keisler, "Model theory" , North-Holland (1973)