Weyl problem

The problem of realizing, in three-dimensional Euclidean space, a regular metric of positive curvature given on a sphere — i.e. the problem of the existence of a regular ovaloid for a prescribed metric. The problem was posed in 1915 by H. Weyl [1]. H. Lewy

in 1937 solved the Weyl problem for the case of an analytic metric: An analytic metric of positive curvature, defined on a sphere, is always realized by some analytic surface in three-dimensional Euclidean space. A complete solution of the Weyl problem was given by the theorem of A.D. Aleksandrov [3] on the realization of a metric of positive curvature by a convex surface, in conjunction with a theorem of A.V. Pogorelov on the regularity of a convex surface with a regular metric. This solution says that a regular metric of class 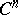, , 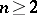of positive Gaussian curvature, defined on a manifold which is homeomorphic to a sphere, can be realized by a closed regular convex surface of class at least 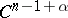, 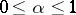. If the metric is analytic, the surface is analytic as well. Pogorelov ([3], Chapt. 6) posed and solved Weyl's problem for the general case of a three-dimensional Riemann surface.

References

[1]	H. Weyl, "Ueber die Bestimmung einer geschlossenen konvexen Fläche durch ihr Linienelement" Vierteljahrschrift Naturforsch. Gesell. Zurich , 3 : 2 (1916) pp. 40–72
[2a]	H. Lewy, "A priori limitations for solutions of Monge–Ampère equations" Trans. Amer. Math. Soc. , 37 (1935) pp. 417–434
[2b]	H. Lewy, "On the non-vanishing of the Jacobian in certain one-to-one mappings" Bull. Amer. Math. Soc. , 42 (1936) pp. 689–692
[3]	A.V. Pogorelov, "Extrinsic geometry of convex surfaces" , Amer. Math. Soc. (1972) (Translated from Russian)

References

[a1]	R. Schneider, "Boundary structure and curvature of convex bodies" J. Tölke (ed.) J.M. Wills (ed.) , Contributions to geometry , Birkhäuser (1979) pp. 13–59
[a2]	L. Nirenberg, "The Weyl and Minkowski problems in differential geometry in the large" Comm. Pure Appl. Math. , 6 (1953) pp. 337–394