Semi-lattice

A commutative idempotent semi-group, that is, a semi-group satisfying the identities 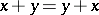and . Every semi-lattice can be turned into a partially ordered set (the partial order 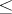is defined by the relation 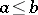if and only if ) in which for any pair of elements there is a least upper bound 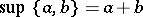. Conversely, every partially ordered set with least upper bounds for every pair of elements is a semi-lattice with respect to the operation 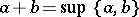. In this case one says that the partially ordered set is an upper semi-lattice (or a join semi-lattice, or a 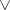-semi-lattice). A lower semi-lattice, also called a meet semi-lattice or a 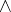-semi-lattice, is dually defined as a partially ordered set in which any two elements have a greatest lower bound.

Comments

A band is a semi-group every element of which is idempotent (cf. also Band of semi-groups) (which is a decomposition of a semi-group into sub-semi-groups forming a band). Thus, an upper (lower) semi-lattice defines a commutative band, and conversely.

References

[a1]	A.H. Clifford, G.B. Preston, "The algebraic theory of semigroups" , 1 , Amer. Math. Soc. (1961) pp. §1.8