Categoricity in cardinality

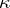
The property of a class of algebraic systems (models) requiring that all systems of cardinality of the class be isomorphic. A first-order theory is said to be categorical in cardinality 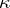if all models of cardinality 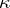of 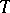are isomorphic. For a countable complete theory 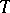, categoricity in countable cardinality (in 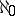) holds if and only if there exists, for any natural number , a finite set 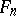of formulas of the language of 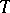with free variables such that any formula of the language with free variables is equivalent in the theory 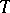to one of the formulas of 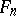. The collection of axioms:

1) ,

2) ,

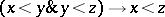3) ,

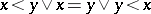4) 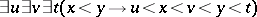, defines a theory 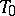of dense linear orderings which is categorical in , but is non-categorical in all uncountable cardinalities. The theory 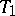of algebraically closed fields of characteristic zero is categorical in all uncountable cardinalities, but is not categorical in 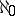. The following general theorem holds: If a first-order countable theory 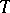is categorical in some uncountable cardinality, then it is categorical in all uncountable cardinalities. This result generalizes to uncountable theories on replacing the condition of uncountable cardinality by cardinality greater than that of 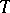. By a quasi-identity one means the universal closure of a formula

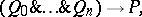where 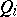and 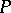are atomic formulas. For countable theories 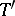which are axiomatized by means of quasi-identities, there is an even smaller distribution of possible categoricities: If such a theory 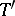is categorical in countable cardinality, then it is categorical in all infinite cardinalities. If one appends to the axiom of the theory 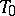axioms for constants :

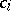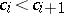) , where 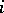runs through the natural numbers, then the theory 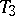so obtained has exactly three countable models (up to isomorphism), since only three cases are possible: the set 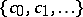has no upper bound, has an upper bound but no least upper bound, or has a least upper bound. If for two countable models 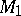and of the theory the same one of the above three cases applies, then 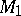is isomorphic to 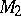. Among theories which are categorical in uncountable cardinalities it is impossible to obtain an analogue of the above example. Thus, if a first-order theory 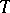is categorical in uncountable cardinality, then the number of countable models of 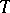(up to isomorphism) is either 1 or infinite.

References

[1]	G.E. Sacks, "Saturated model theory" , Benjamin (1972)
[2]	E.A. Palyutin, "Description of categorical quasivarieties" Algebra and Logic , 14 (1976) pp. 86–111 Algebra i Logika , 14 (1975) pp. 145–185
[3]	S. Shelah, "Categoricity of uncountable theories" , Proc. Tarski Symp. , Proc. Symp. Pure Math. , 25 : 2 (1974) pp. 187–203

Comments

The definition of a quasi-identity can also be found in Algebraic systems, quasi-variety of.

The "general theorem" mentioned in the text was conjectured by J. Łoś [a1], to whom the term "categoricity" is due, and proved by M.D. Morley [a2].

References

[a1]	J. Łoś, "On the categoricity in power of elementary deductive systems and some related problems" Colloq. Math. , 3 (1954) pp. 58–62
[a2]	M. Morely, "Categoricity in power" Trans. Amer. Math. Soc. , 114 (1965) pp. 514–538
[a3]	C.C. Chang, H.J. Keisler, "Model theory" , North-Holland (1973)
[a4]	S. Shelah, "Classification theory and the number of non-isomorphic models" , North-Holland (1978)