Density theorems

The general name for theorems that give upper bounds for the number of zeros of Dirichlet -functions

where and is a character modulo , in the rectangle , . In the case 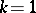, one gets density theorems for the number of zeros of the Riemann zeta-function

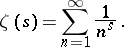The density theorems for 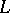-functions with 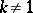are more complicated than those for the Riemann zeta-function. As and increase, one obtains bounds depending on these parameters. The parameter plays a decisive part in applications.

The significance of density theorems is evident from the relations enabling one to estimate the residual term in the formula for the number of prime numbers belonging to an arithmetic progression , , , 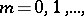and not exceeding , as a function of .

Since does not increase with and 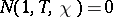, the purpose of density theorems is to obtain bounds that converge most rapidly to zero as . In turn, these bounds are substantially supplemented by results on the absence of zeros for Dirichlet 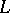-functions in neighbourhoods of the straight line 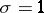, obtained using the Hardy–Littlewood–Vinogradov circle method. In this way it has been possible to obtain strong bounds for the amount of even numbers that cannot be represented as the sum of two prime numbers.

Yu.V. Linnik obtained the first density theorems providing bounds for 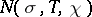for an individual character 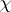and averaged bounds over all characters modulo , given . Subsequent substantial improvements of density theorems were obtained by A.I. Vinogradov and E. Bombieri, who used bounds on averaged over all moduli and over all primitive characters modulo , given 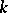, in proving a theorem on the average distribution of prime numbers in arithmetic progressions (for ). The Vinogradov–Bombieri theorem enables one to replace the generalized Riemann hypothesis in various classical problems in additive number theory. There are also various other improvements of density theorems.

References

[1]	K. Prachar, "Primzahlverteilung" , Springer (1957)
[2]	H. Davenport, "Multiplicative number theory" , Springer (1980)
[3]	A.F. Lavrik, "A survey of Linnik's large sieve and the density theory of zeros of 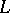-functions" Russian Math. Surveys , 35 : 2 (1980) pp. 63–76 Uspekhi Mat. Nauk , 35 : 2 (1980) pp. 55–65

Comments

For extra references see Density method. Cf. also Distribution of prime numbers.

For the Vinogradov–Bombieri theorem see Density hypothesis.

References

[a1]	A. Ivic, "The Riemann zeta-function" , Wiley (1985)