McKay-Alperin conjecture
Let  be a finite group. For any prime number
be a finite group. For any prime number  , let
, let  be the number of irreducible complex characters of
be the number of irreducible complex characters of  with degree prime to
with degree prime to  (cf. also Character of a group). The simplest form of the McKay–Alperin conjectures asserts that
(cf. also Character of a group). The simplest form of the McKay–Alperin conjectures asserts that
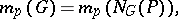 |
where  is a Sylow
is a Sylow  -subgroup of
-subgroup of  and
and  is its normalizer (cf. also Sylow subgroup;
is its normalizer (cf. also Sylow subgroup; 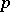 -group; Normalizer of a subset). J. McKay [a2] first suggested this might be true when
-group; Normalizer of a subset). J. McKay [a2] first suggested this might be true when 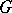 is a simple group. J.L. Alperin [a1] observed that it is probably true for all finite groups.
is a simple group. J.L. Alperin [a1] observed that it is probably true for all finite groups.
Alperin also made a more general conjecture, involving characters in 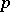 -blocks. (See Brauer first main theorem for notation and definitions.) Let
-blocks. (See Brauer first main theorem for notation and definitions.) Let 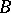 be a
be a 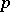 -block of
-block of 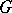 with defect group
with defect group  (cf. Defect group of a block), and let
(cf. Defect group of a block), and let  be an irreducible character (cf. also Irreducible representation) belonging to
be an irreducible character (cf. also Irreducible representation) belonging to 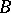 . Let
. Let 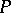 be a Sylow
be a Sylow  -subgroup of
-subgroup of 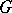 . By a theorem of R. Brauer,
. By a theorem of R. Brauer,  divides the degree
divides the degree  . The character
. The character 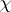 is said to have height zero if the largest power of
is said to have height zero if the largest power of 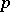 dividing
dividing 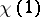 is
is 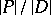 . The more general Alperin conjecture asserts that the number of irreducible characters of height zero in
. The more general Alperin conjecture asserts that the number of irreducible characters of height zero in 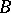 is equal to the number of irreducible characters of height zero in the unique block of
is equal to the number of irreducible characters of height zero in the unique block of 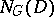 sent to
sent to 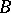 by the Brauer correspondence.
by the Brauer correspondence.
The conjectures are still not proved (1998), but the evidence in their favour is very strong.
References
| [a1] | J.L. Alperin, "The main problem of block theory" W.R. Scott (ed.) F. Gross (ed.) , Proc. Conf. Finite Groups (Park City, Utah, 1975) , Acad. Press (1976) |
| [a2] | J. McKay, "Irreducible representations of odd degree" J. Algebra , 20 (1972) pp. 416–418 |
McKay-Alperin conjecture. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=McKay-Alperin_conjecture&oldid=32403