Radical

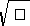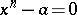A radical is the mathematical symbol (modified Latin ). It denotes the extraction of a root, that is, the solution of a two-term algebraic equation of the form . The symbol 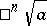denotes one of the roots of this equation.

The problem of the solution of algebraic equations over the complex field 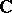in radicals is to express the roots of an algebraic equation (with complex coefficients) in terms of its coefficients using a finite number of additions, multiplications, divisions, raising to a power, and root extractions. Equations of degree higher than 4 cannot, in general, be solved by radicals (see Galois theory).

The radical symbol is also used for the notation of the radical of an ideal.

In certain algebraic systems the radical is a concept connected with that of a radical property. The first examples of radicals arose in the theory of associative rings (see the more detailed article Radical of rings and algebras). The construction of a general theory of radicals was begun by S. Amitsur and A.G. Kurosh. The theory of radicals can be developed in any category of algebraic systems having certain necessary properties (for example, in the category of multi-operator groups). Many questions of the theory of radicals have been studied within category theory. See also Radical of a group; Radical in a class of semi-groups; Quasi-regular radical.