Rank of a group

(general and special rank of a group)

A notion from group theory. A group 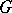has finite general rank if is the minimal number such that any finitely-generated subgroup of is contained in a subgroup having generators 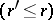. A group has finite special rank if is the minimal number such that any finitely-generated subgroup of has a system of generators of at most elements. If no such finite number exists, then the general (special) rank of the group is said to be infinite.

The general rank of a group is smaller than or equal to its special rank. There are groups with finite general rank (even equal to two) and infinite special rank. Such is, for instance, the countable symmetric group. For an Abelian group the general and special rank coincide with its Prüfer rank (see Abelian group).

References

[1]	A.G. Kurosh, "The theory of groups" , 1–2 , Chelsea (1955–1956) (Translated from Russian)