Parabolic point
From Encyclopedia of Mathematics
A point on a regular surface at which the osculating paraboloid degenerates into a parabolic cylinder. At a parabolic point the Dupin indicatrix is a pair of parallel straight lines, the Gaussian curvature is equal to zero, one of the principal curvatures (cf. Principal curvature) vanishes, and the coefficients of the second fundamental form satisfy the equation
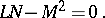 |
Comments
References
| [a1] | W. Klingenberg, "A course in differential geometry" , Springer (1978) pp. 50–51 (Translated from German) |
| [a2] | R.S. Millman, G.D. Parker, "Elements of differential geometry" , Prentice-Hall (1977) pp. 132 |
How to Cite This Entry:
Parabolic point. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Parabolic_point&oldid=31651
Parabolic point. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Parabolic_point&oldid=31651
This article was adapted from an original article by D.D. Sokolov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article