Homothety

A transformation of Euclidean space with respect to a certain point that brings each point in a one-to-one correspondence with a point on the straight line in accordance with the rule

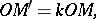where is a constant number, not equal to zero, which is known as the homothety ratio. The point is said to be the centre of the homothety. If , the points and 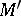lie on the same ray; if , on different sides from the centre. The point corresponds to itself. A homothety is a special case of a similarity. Two figures called homothetic (similar or similarly situated) if each one consists of points obtained from the other figure by a homothety with respect to some centre.

Simplest properties of a homothety. A homothety with is a one-to-one mapping of the Euclidean space onto itself, with one fixed point. If , the homothety is the identity transformation. A homothety maps a straight line (a plane) passing through its centre into itself; a straight line (a plane) not passing through its centre into a straight line (a plane) parallel to it; the angles between straight lines (planes) are preserved under this transformation. Under a homothety segments are mapped into parallel segments with a length which is times the original length, i.e. a homothety is a contraction (expansion) of the Euclidean space at the point . Under a homothety a sphere is mapped into another sphere, and the centre of the former is mapped to the centre of the latter.

A homothety is most often specified (geometrically) by the homothety centre and a pair of corresponding points or by two pairs of corresponding points. A homothety is an affine transformation with one (and only one) fixed point.

In -dimensional Euclidean space a homothety leaves the set of all 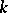-dimensional subspaces invariant, .

A homothety is defined in a similar manner in pseudo-Euclidean spaces. A homothety in Riemannian spaces and in pseudo-Riemannian spaces is defined as a transformation that transforms the metric of the space into itself, up to a constant factor. The set of homotheties forms a Lie group of transformations, and the -parameter homothety group of a Riemannian space contains the -parameter normal subgroup of displacements.

Comments

A homotopy is also called a central dilatation (cf. also Dilatation).

References

[a1]	M. Berger, "Geometry" , 1–2 , Springer (1987) (Translated from French)
[a2]	H.S.M. Coxeter, "Introduction to geometry" , Wiley (1961)
[a3]	E. Artin, "Geometric algebra" , Interscience (1957)