Astroid

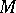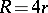A plane algebraic curve of order six, described by a point on a circle of radius rolling on the inside of a circle of radius ; a hypocycloid with module 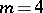. Its equation in orthogonal Cartesian coordinates is

and a parametric representation is

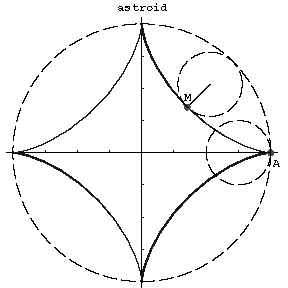
Figure: a013540a

There are four cusps (see Fig.). The length of the arc from the point 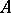is

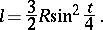The length of the entire curve is . The radius of curvature is

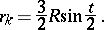The area bounded by the curve is

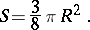The astroid is the envelope of a family of segments of constant length, the ends of which are located on two mutually perpendicular straight lines. This property of the astroid is connected with one of its generalizations — the so-called oblique astroid, which is the envelope of the segments of constant length with their ends located on two straight lines intersecting at an arbitrary angle.

References

[1]	A.A. Savelov, "Planar curves" , Moscow (1960) (In Russian)

References

[a1]	J.D. Lawrence, "A catalog of special plane curves" , Dover, reprint (1972)
[a2]	E.A. Lockwood, "A book of curves" , Cambridge Univ. Press (1961)