Cauchy-Riemann conditions
d'Alembert–Euler conditions
Conditions that must be satisfied by the real part 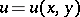 and the imaginary part
and the imaginary part 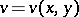 of a complex function
of a complex function 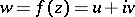 ,
,  , for it to be monogenic and analytic as a function of a complex variable.
, for it to be monogenic and analytic as a function of a complex variable.
A function 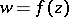 , defined in some domain
, defined in some domain 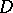 in the complex
in the complex  -plane, is monogenic at a point
-plane, is monogenic at a point  , i.e. has a derivative at
, i.e. has a derivative at  as a function of the complex variable
as a function of the complex variable  , if and only if its real and imaginary parts
, if and only if its real and imaginary parts  and
and  are differentiable at
are differentiable at  as functions of the real variables
as functions of the real variables  and
and 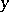 , and if, moreover, the Cauchy–Riemann equations hold at that point:
, and if, moreover, the Cauchy–Riemann equations hold at that point:
 | (1) |
If the Cauchy–Riemann equations are satisfied, then the derivative  can be expressed in any of the following forms:
can be expressed in any of the following forms:
 |
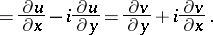 |
A function  , defined and single-valued in a domain
, defined and single-valued in a domain  , is analytic in
, is analytic in  if and only if its real and imaginary parts are differentiable functions satisfying the Cauchy–Riemann equations throughout
if and only if its real and imaginary parts are differentiable functions satisfying the Cauchy–Riemann equations throughout  . Each of the two functions
. Each of the two functions  and
and  of class
of class  satisfying the Cauchy–Riemann equations (1) is a harmonic function of
satisfying the Cauchy–Riemann equations (1) is a harmonic function of  and
and  ; the conditions (1) constitute conjugacy conditions of these two harmonic functions: Knowing one of them, the other may be found by integration.
; the conditions (1) constitute conjugacy conditions of these two harmonic functions: Knowing one of them, the other may be found by integration.
The conditions (1) are valid for any two orthogonal directions  and
and  , with the same mutual orientations as the
, with the same mutual orientations as the  - and
- and  -axes, in the form:
-axes, in the form:
 |
For example, in polar coordinates  , for
, for  :
:
 |
Defining the complex differential operators by
 |
one can rewrite the Cauchy–Riemann equations (1) as
 |
Thus, a differentiable function  of the variables
of the variables  and
and  is an analytic function of
is an analytic function of  if and only if
if and only if  .
.
For analytic functions of several complex variables  ,
, 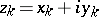 ,
,  , the Cauchy–Riemann equations constitute a system of partial differential equations (overdetermined when
, the Cauchy–Riemann equations constitute a system of partial differential equations (overdetermined when  ) for the functions
) for the functions
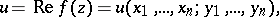 |
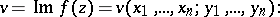 |
 | (2) |
or, in terms of the complex differentiation operators:
 |
Each of the two functions  and
and  of class
of class  satisfying the conditions (2) is a pluriharmonic function of the variables
satisfying the conditions (2) is a pluriharmonic function of the variables  and
and  (
( ). When
). When  the pluriharmonic functions constitute a proper subclass of the class of harmonic functions. The conditions (2) are conjugacy conditions for two pluriharmonic functions
the pluriharmonic functions constitute a proper subclass of the class of harmonic functions. The conditions (2) are conjugacy conditions for two pluriharmonic functions  and
and  : Knowing one of them, one can determine the other by integration.
: Knowing one of them, one can determine the other by integration.
The conditions (1) apparently occurred for the first time in the works of J. d'Alembert [1]. Their first appearance as a criterion for analyticity was in a paper of L. Euler, delivered at the Petersburg Academy of Sciences in 1777 [2]. A.L. Cauchy utilized the conditions (1) to construct the theory of functions, beginning with a memoir presented to the Paris Academy in 1814 (see [3]). The celebrated dissertation of B. Riemann on the fundamentals of function theory dates from 1851 (see [4]).
References
| [1] | J. d'Alembert, "Essai d'une nouvelle théorie de la résistance des fluides" , Paris (1752) |
| [2] | L. Euler, Nova Acta Acad. Sci. Petrop. , 10 (1797) pp. 3–19 |
| [3] | A.L. Cauchy, "Mémoire sur les intégrales définies" , Oeuvres complètes Ser. 1 , 1 , Paris (1882) pp. 319–506 |
| [4] | "Grundlagen für eine allgemeine Theorie der Funktionen einer veränderlichen komplexen Grösse" H. Weber (ed.) , Riemann's gesammelte math. Werke , Dover, reprint (1953) pp. 3–48 (Dover, reprint, 1953) |
| [5] | A.I. Markushevich, "Theory of functions of a complex variable" , 1 , Chelsea (1977) pp. Chapt. 1 (Translated from Russian) |
| [6] | B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1976) pp. 1, Chapt. 1; 2, Chapt. 1 (In Russian) |
Comments
References
| [a1] | L.V. Ahlfors, "Complex analysis" , McGraw-Hill (1979) pp. 24–26 |
Cauchy-Riemann conditions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cauchy-Riemann_conditions&oldid=31183